Did you know using an abacus can improve your child's intelligence? According to research, using an Abacus can improve problem-solving skills in kids by impacting their intelligence.
Besides problem-solving skills, there are more cognitive benefits to using an abacus at an early age. But, I am guessing it's a not brand-new information for you.
Is it? As a matter of fact, most of us are aware that the abacus has incredible benefits but only a handful of us know how to teach abacus to kids. In other words, many don't know how to use abacus?
Dear fellow parents, I understand your concern. Therefore, here, I am going to explain how to use an abacus for solving basic math operations.
Using an Abacus Made Easy
1. An Abacus can be used in different Ways
The abacus is versatile—helping kids learn numbers and even perform advanced calculations like cube roots. It makes math fun and effective.

I will tell you how to use abacus step-by step. First, I will take you through how to teach counting and place value using an abacus. Then, I will explain how to conduct basic math operations, such as addition, subtraction, multiplication, division.
Counting
Surveys show that abacus improves memory, focus, and cognitive skills. That’s why it’s used in preschools, primary schools, and even for visually impaired children.
Its most popular use? Counting! Let’s see how to count on an abacus.
Method 1
Counting 1 to 10 on Abacus Step-by-Step Process
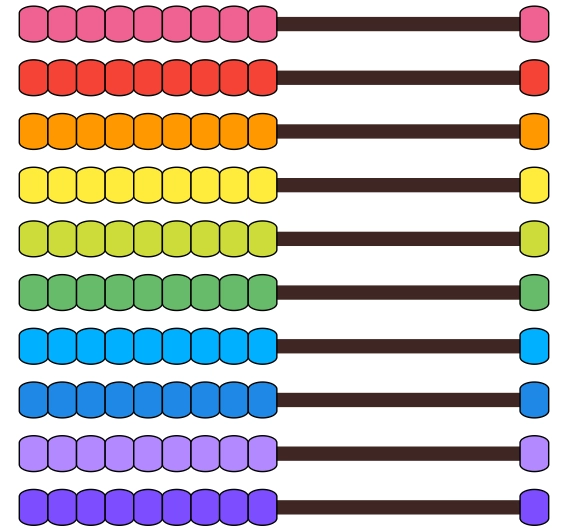
In the beginning, arrange the beads at the left-hand side of the abacus.
Now, separate one bead on each row and push it towards the right corner.
Next, count the beads one by one. As there is one bead on each row, the total count is ten. Dear reader, this is one method you can use to introduce counting to your little child on an abacus.
Dear reader, this is one method you can use to introduce counting to your little child on an abacus.
Wondering what are the benefits of Abacus?

Method 2
Learn 1 to 100 there are 100 beads on the regular Abaci that are used at schools and homes. You can teach your child how to count by going through the beads one by one.
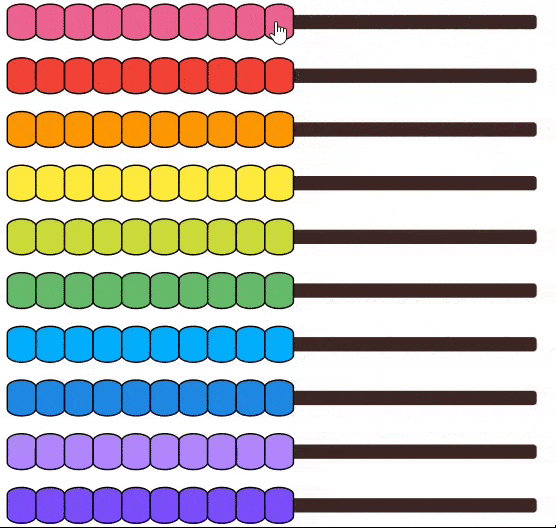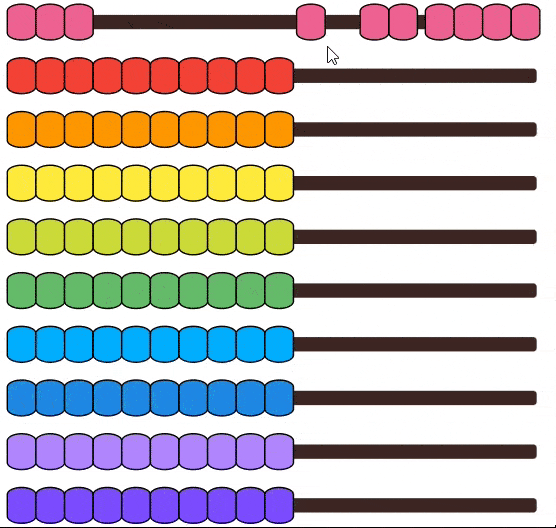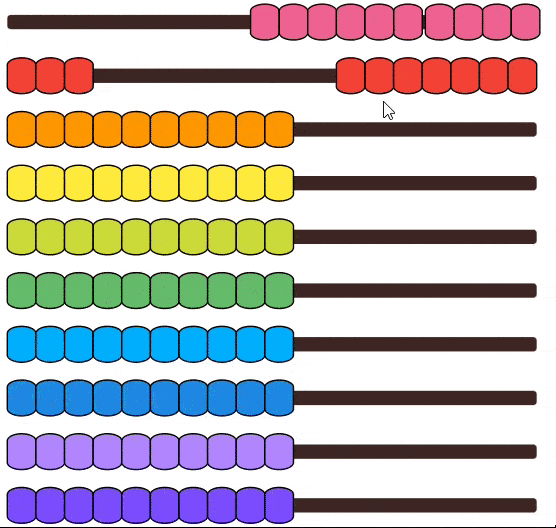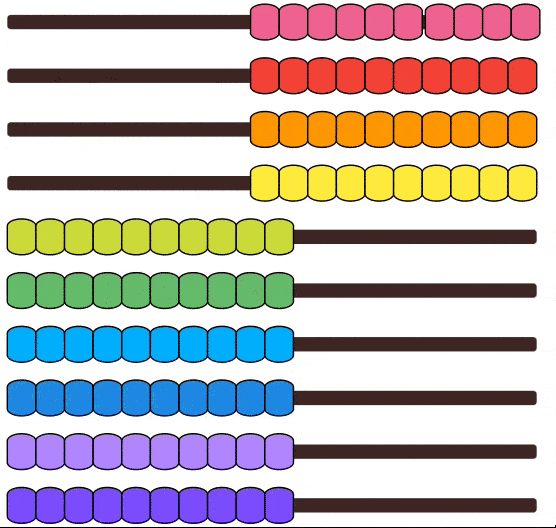
Counting 1 to 100 on Abacus Step-by-Step Process.
Push all the beads to the left before starting to count.
Now, begin by sliding 1 bead at a time on the first row from left to right.
Keep counting till you're out of beads and the count is 10.
Then, repeat the same process to count 11 to 20 on the second row, and 20 to 30 in the third row, and so on.
Continue counting like this row after row till you reach the last one and the counting is 100. It is the most traditional and common method of teaching counting on the abacus. Also, it's the most effective way to remember numbers.
It is the most traditional and common method of teaching counting on the abacus. Also, it's the most effective way to remember numbers. If your little one still struggles to count, then, watch this video to help them understand.
Counting numbers in patterns.
https://enthu.com/blog/abacus/benefits-of-abacus/

2. Teaching Place Values
I understand that teaching place values can be one of the most difficult levels in beginner’s mathematics. But, worry no more! If you know how to use abacus, you problem's solved.
The Abacus is the most efficient tool for teaching place values. Therefore, I suggest you try the counting frame.
Method 1
First and foremost, you need to explain to your child that 10 beads in ones row = 1 bead in the tens row (on an abacus).
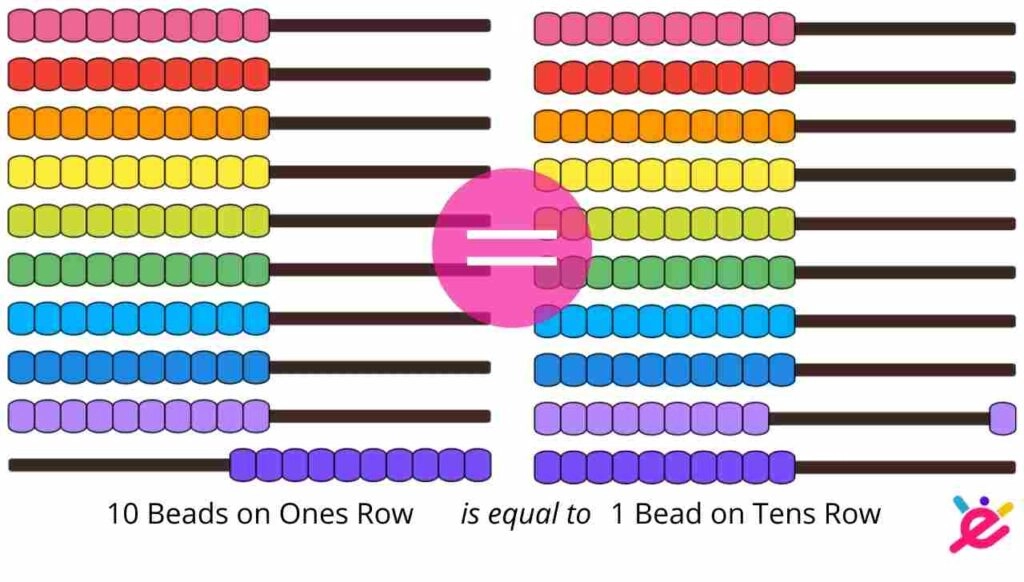
Understanding Place Values on Abacus Step-by-Step Process
Begin by sliding 10 beads towards the right on the last row (as shown in the first Abacus in the picture). Now, ask the child to count.
Next, keep all the beads on the left but only 1 bead on the right on second last row which is tens row.
Now, explain how 10 beads on ones row is equal to 1 bead on the tens row. Refer to the picture while explaining.
Next, arrange 10 beads in 10 rows and show how 10 tens become 1 hundred. Take help of the counting frame and follow the above methods. I am sure, in no time, your child will understand how 10
Take help of the counting frame and follow the above methods. I am sure, in no time, your child will understand how 10 beads on the hundredths' row = 1 bead on thousandths' row.
Method 2
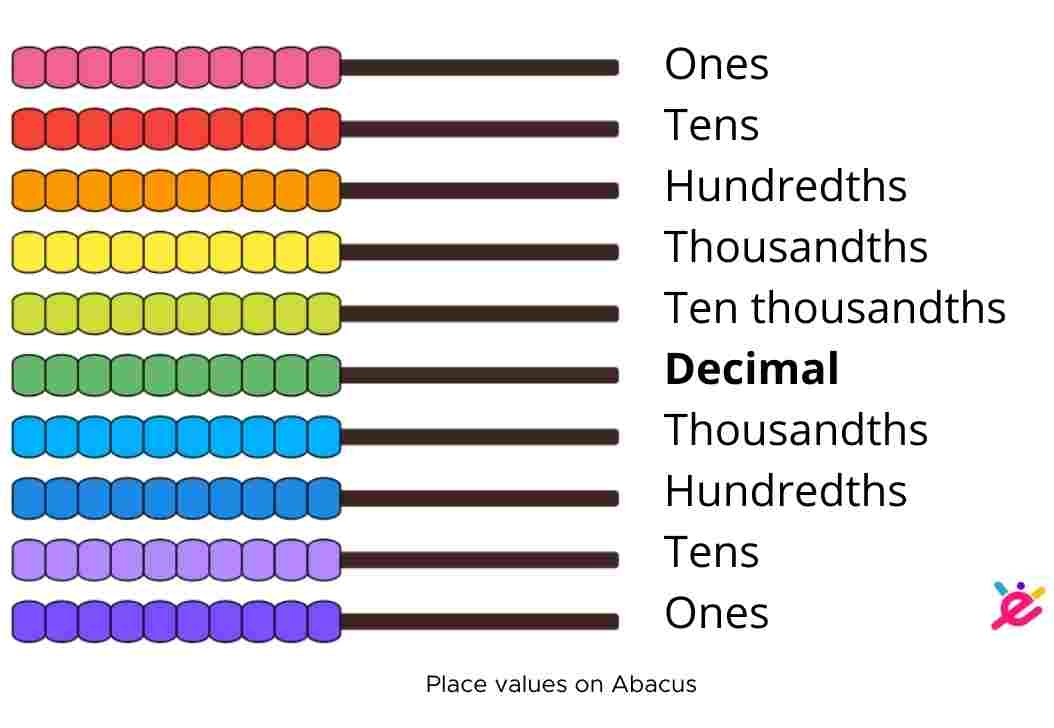
Step-by-Step Process
Start with the last row. Explain to your child that the last row stands for ones–1, 2, 3…and so on.
The second row stands for 10, 20, 30…so on.
The third row stands for 100, 200, 300, 400, and so on.
Thus, as we go up, the place value keeps on increasing until we reach 5th row from below, which is decimal row.
As shown in the picture, the top rows also follow similar place values. The top row is the ones row, next is the tens row, the third row is the hundredths row, and the fourth row is the ten thousandth row.
Dear Readers, remember, you can change the place values of the rows as your convenience. For instance, if you are representing 1 million on the abacus than you need to give up the decimal-row, and keep increasing the place values of the rows till you reach 7th row from the bottom.
However, you should the follow the above place value system on abacus to solve basic math operations. Why? Because it's convenient and easy for kids.
3. Represent Bigger Number
Once you think your child understands place values, the next step is to teach them how to represent bigger numbers on the abacus.
Example: represent 58 on an abacus.
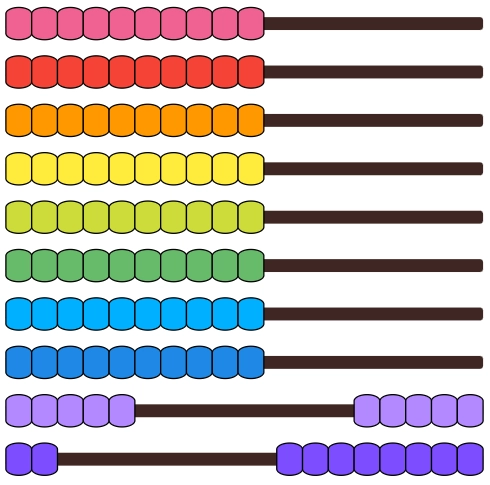
Step-by-Step Process
Which digit is in the tens’ place. 5, right? So, put 5 beads towards the left side on the tens’ row (second last row).
Next, ask them about the digit in the ones’ place. Then, push 8 beads on the ones’ row (bottom row) towards the left side. There you have 58 on the abacus. Once your child is clear with place values, ask them to represent thousand and millions on.
Once your child is clear with place values, ask them to represent thousand and millions on.
Try to represent 50043024 on Abacus. First, convert the number in words; 50043024 is fifty million forty-three thousand twenty-four. If you're finding it difficult, just try to keep the place value of the rows in mind.
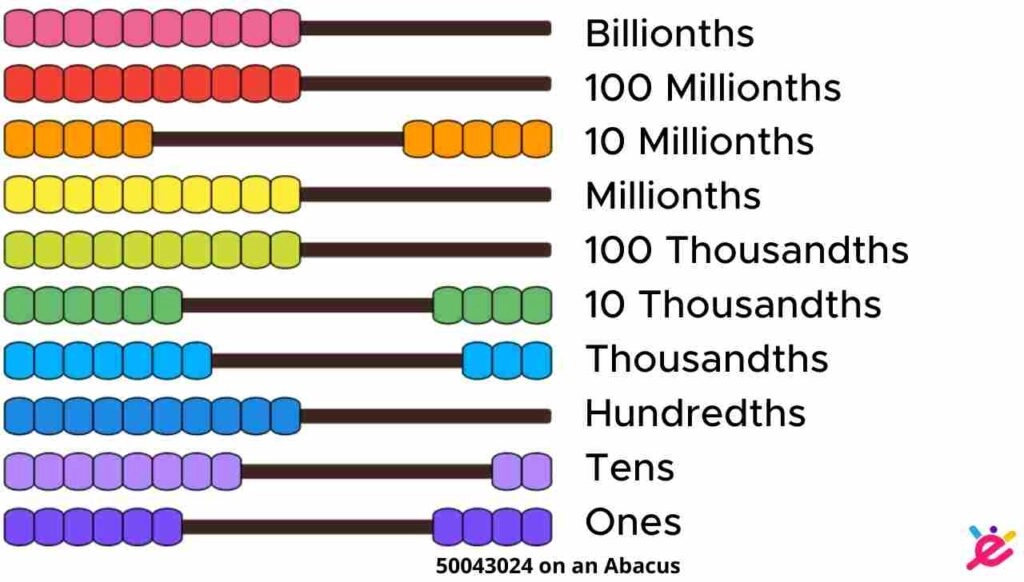

4. Addition
Did you know Abaci is used in various mind development programs?
Yep, you read it right. Using abaci has shown significant results for mental math training programs. Dear reader, although addition seems to be a basic math operation, it's, in fact, a stepping stone towards learning complex calculations of mental mathematics. So, let's go ahead and learn how to use an Abacus for addition.
Method 1
Let’s begin with the easiest addition problems. Let’s say we are adding
Let’s begin with the easiest addition problems. Let’s say we are adding 2 + 2.
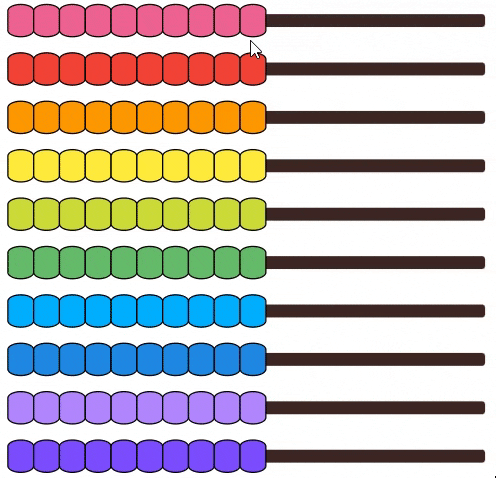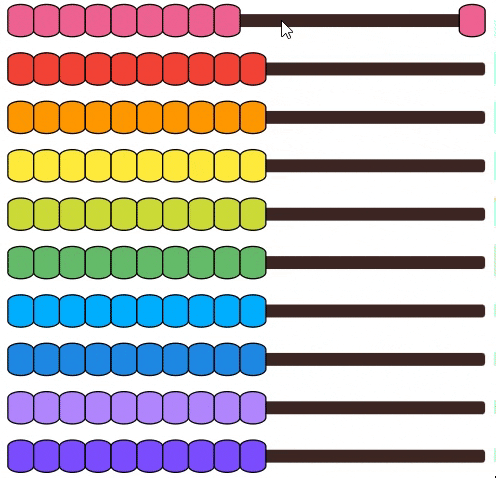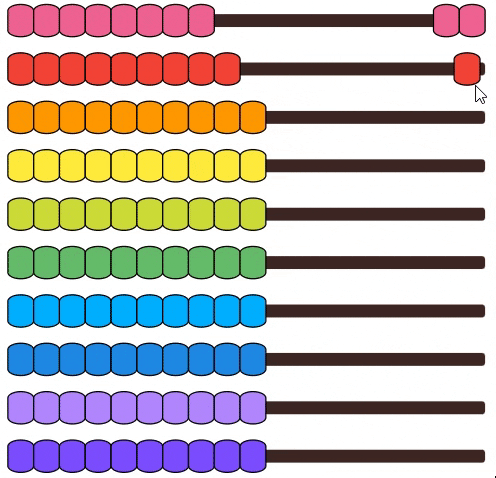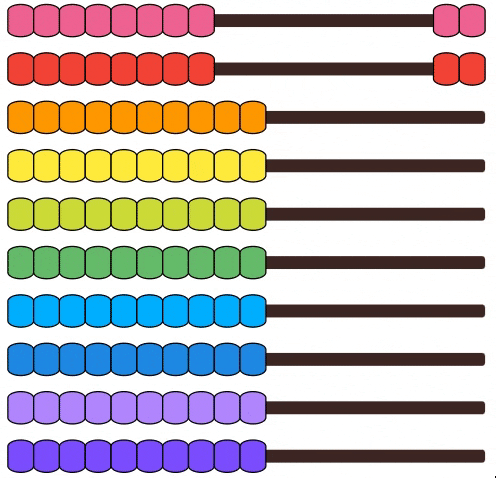
2+2=4 on Abacus Step-by-Step Process
Begin by pushing all the beads towards the left side.
Now, take two beads on the first row and push them towards the right side.
Next, ask your child to repeat the same step on the second row. What do we have?
We have 4 beads.
It's traditional yet the easiest method of adding on an abacus.
You can try this if your child is a preschooler or in kindergarten.
Method 2
The 10 Method “The 10 method” is an
“The 10 method” is an addition strategy performed on an abacus. It's crucial because it is one of the first steps towards learning mental math. Let’s look at an example. Let’s say you want to add 9 + 4.
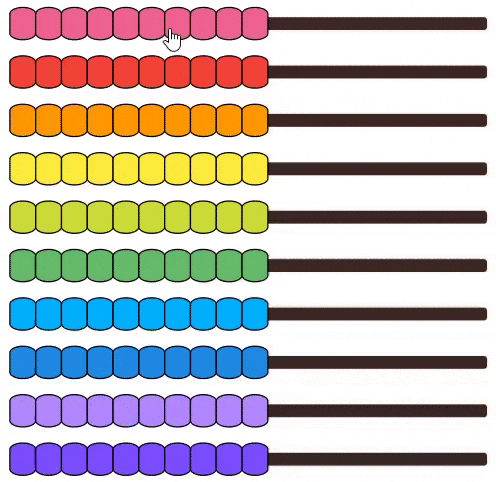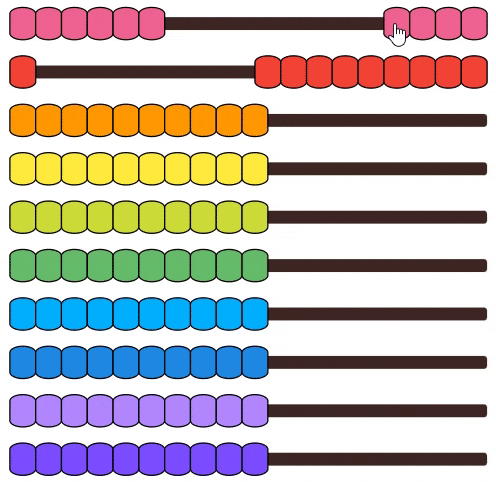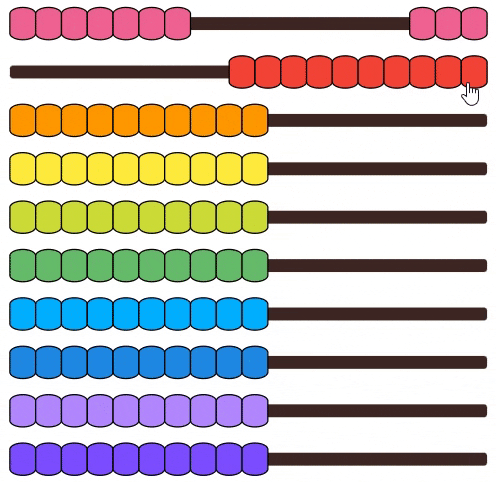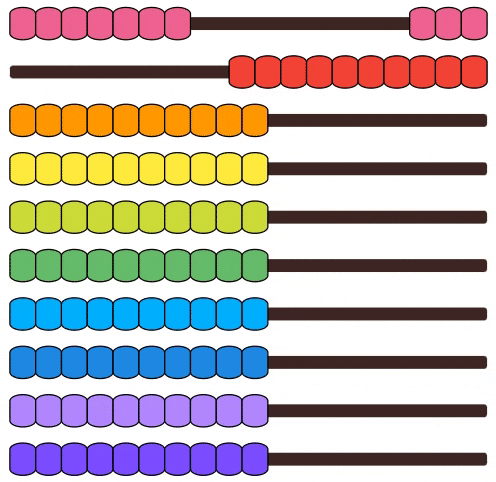
Step-by-Step Process
So, first, arrange 4 and 9 beads (towards the right) on the first two rows of the counting frame.
Then, take a bead from the 4 beads row and push towards the left while adding another bead to the 9 beads row. Now, what happens?
The 9 beads become a full 10, and the 4 become a 3.
This is how you can teach your child 9 + 4 = 10 + 3 = 13. Once your child practices this method a few times, encourage them to picture the beads in their minds. You can also give them a problem to solve without using the beads. I have given an example for kids between 3 to 6 years old. You can increase the level according to your child's standard.
Once your child practices this method a few times, encourage them to picture the beads in their minds. You can also give them a problem to solve without using the beads.
I have given an example for kids between 3 to 6 years old. You can increase the level according to your child's standard.
Method 3
The Two 5s Method To understand this method, try adding
To understand this method, try adding 7+8.
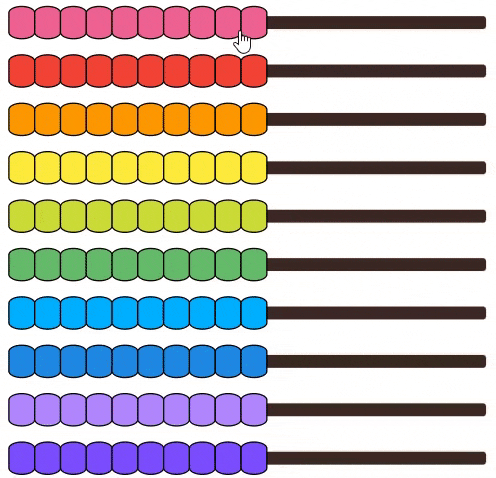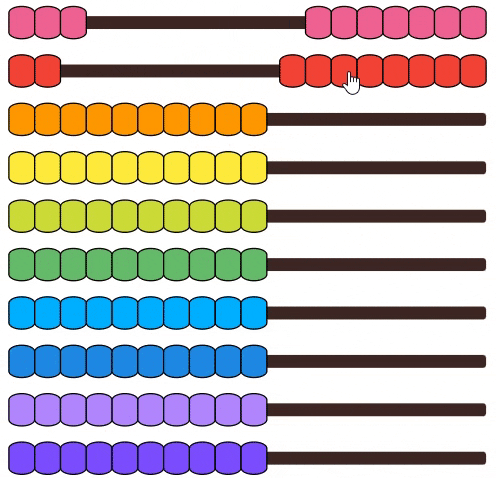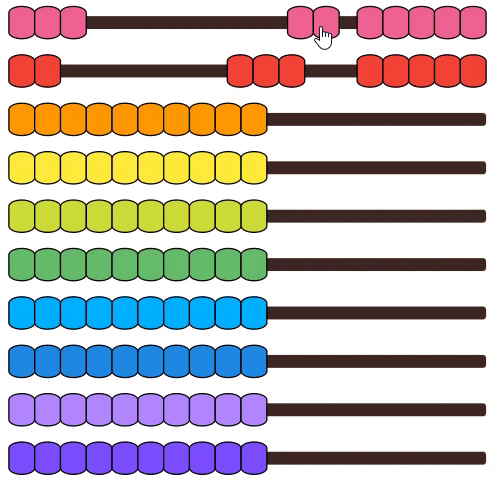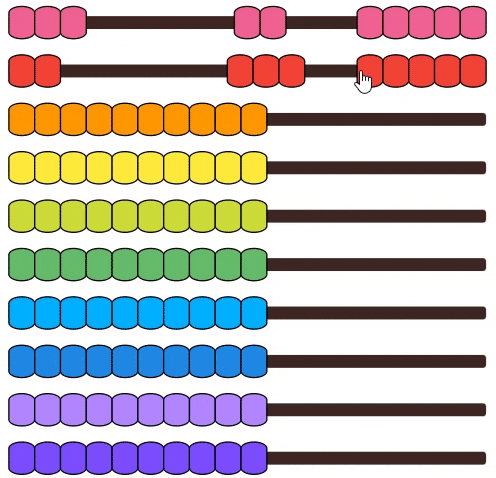
The Two 5s Method: 7+8 Step-by-Step Process
Begin by arranging 7 and 8 beads on the first two rows.
Once you have done that, separate the first 5 beads of each row, making it two 5 and becoming a complete 10.
Now, the remaining beads add up to 5, giving 15.
Method 4
Adding Double Digit Numbers Let's try
Let's try to add 56+27.
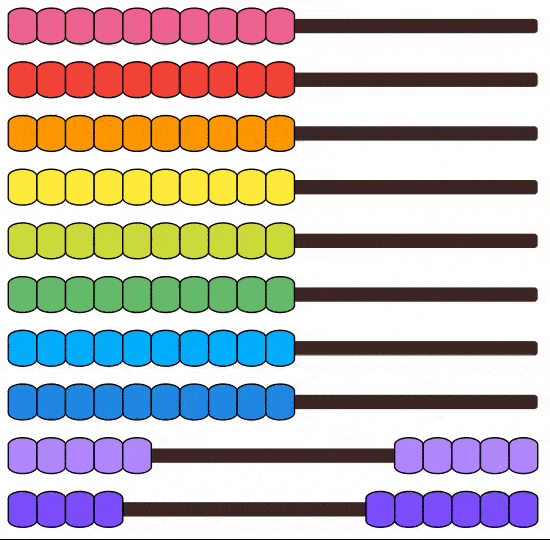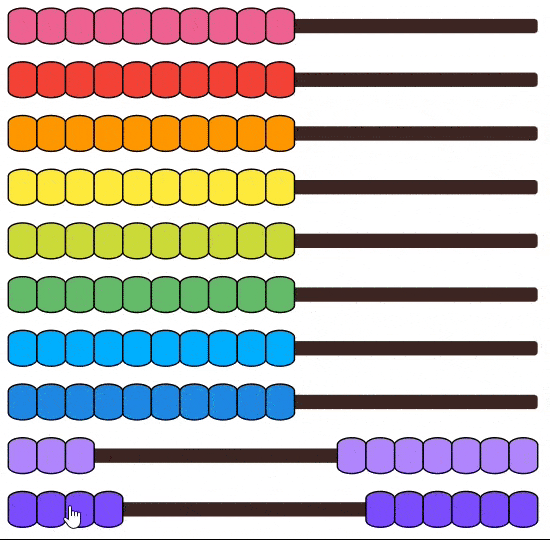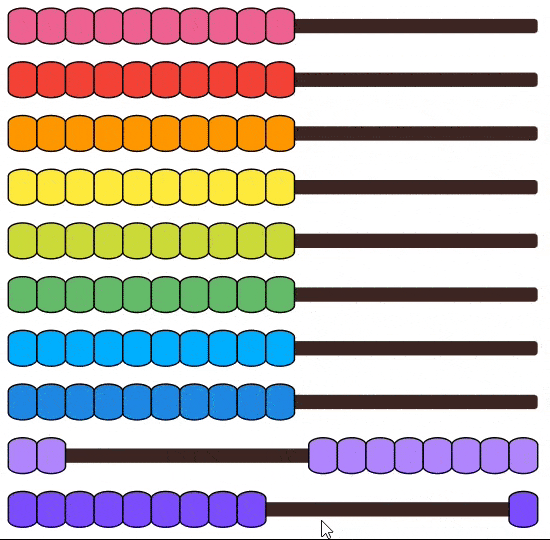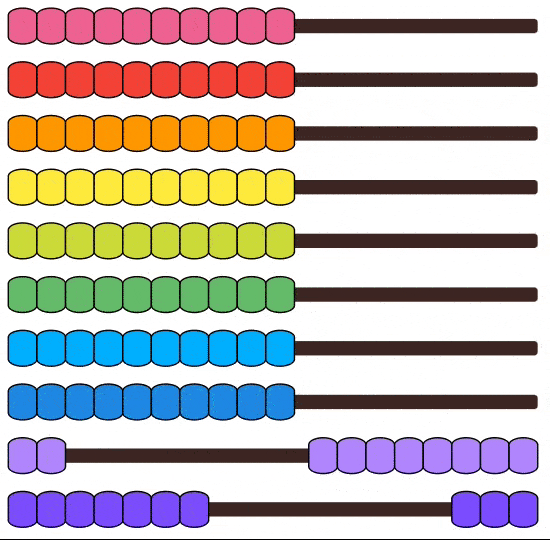
Step-by-Step Process
Begin by representing 56 on abacus, which means 5 beads on tens-row and 6 beads on ones-row.
Next, we need to add 27. So, ask the abacus learner what is on the on tenth place? It's 2, so, we add 2 beads on the tens-row.
Now, we begin to add 7 beads on ones-row. So, keep pushing beads from left to right on the ones-row.
After adding 4 beads when you're out of beads on the ones-row, push all the beads towards the left.
In exchange, you can add 1 bead on the tens row. (Because 10 beads on ones-row=1 bead on tens-row.)
Now, we slide remaining 3 beads to the left on the ones-row.
With 8 beads on tens-row and 3 beads on ones-row, the answer is 83
Method 5
Adding Triple-Digit Numbers Let's try to
Let's try to add 789 + 105.
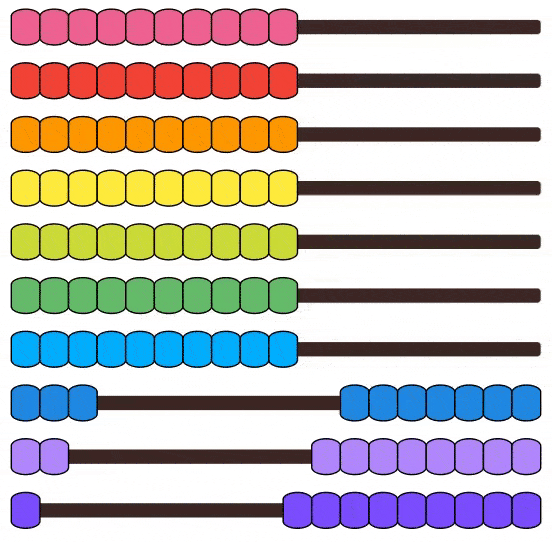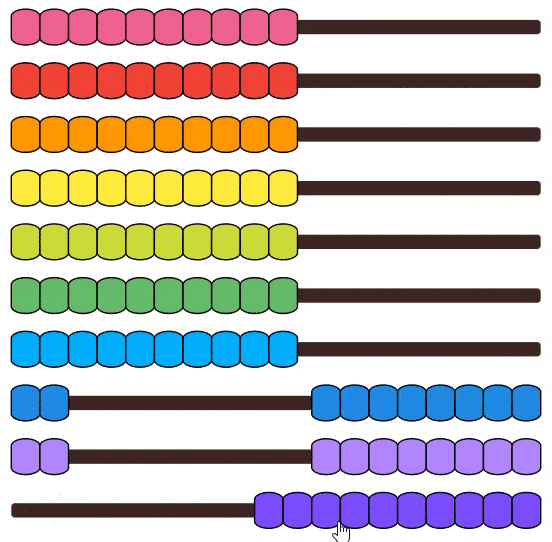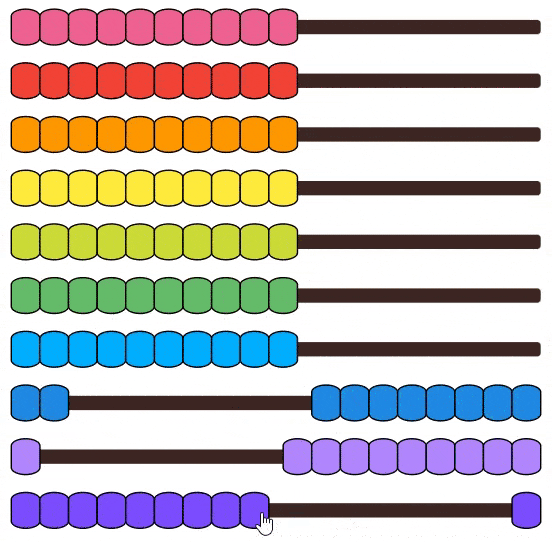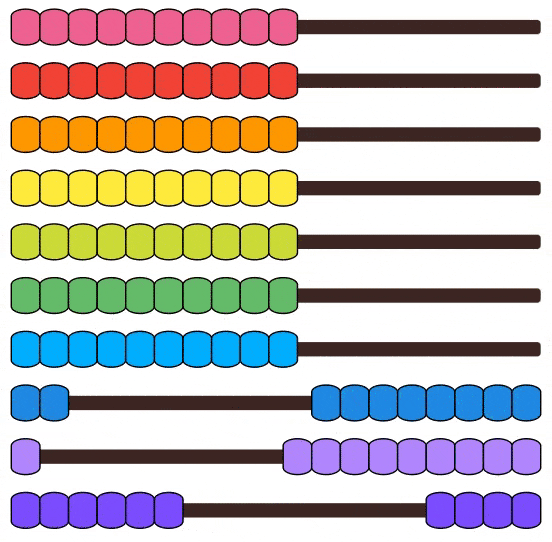
Three Digit Addition Step-by-Step Process
Begin by representing 789 on abacus, which means 7 beads on hundredths-row, 8 beads on tens-row, and 9 beads on ones-row.
Next, we need to add 105. So, ask the abacus learner about the digits and their place values.
Now, we add 1 bead on hundredths-row because it's 1 on the hundredth place in 105.
We don't add any bead from left to right on tenths-row because it's 0 on tenth place in the number 105.
Now, as 5 is on the ones place, we need to add 5 beads on the ones-row.
After adding 1 bead when you're out of beads on the ones-row, push all the beads towards the left.
In exchange, add 1 bead on the tens row. (Because 10 beads on ones-row=1 bead on tens-row.)
Now, we slide remaining 4 beads to the left on the ones-row.
With 8 beads on hundredth-row, 9 beads on tenth-row and 4 beads on ones-row, the answer is 894.
5. Subtraction
Method 1
Subtracting Single-Digit Numbers Always begin by teaching them how to subtract single-digit numbers on the abacus. Let's try 8 - 5.
Always begin by teaching them how to subtract single-digit numbers on the abacus. Let's try 8 - 5.
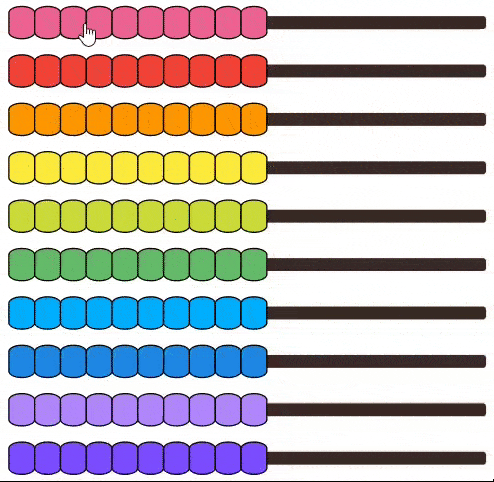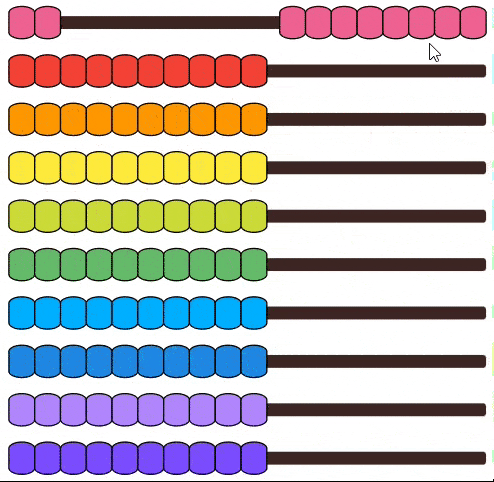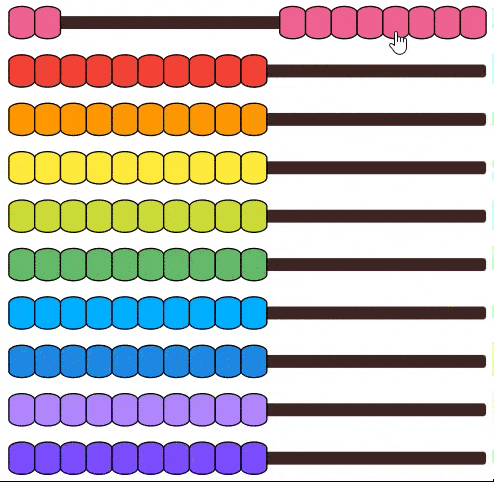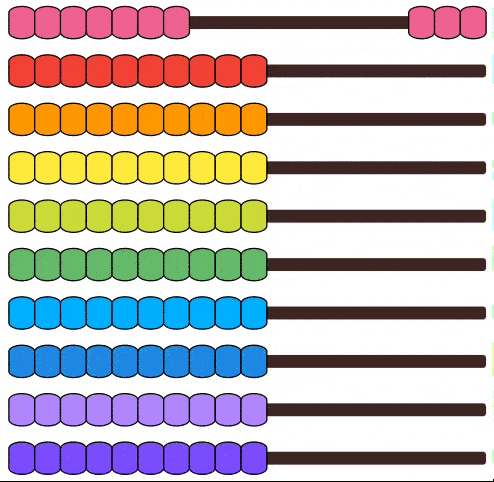
Single Digit Subtraction Step-by-Step Process
First, push all the beads on the first row towards your left-hand side.
Next, ask your child to count 8 beads and push them towards the right-hand side.
As we are subtracting 5, ask your child to count 5 beads from the 8 and push them back towards the left-hand side.
We are left with 3 beads; therefore, the answer is 3.
Method 2
Subtracting Double-Digit Numbers on Abacus Let's say we are trying to solve
Let's say we are trying to solve 67-32.
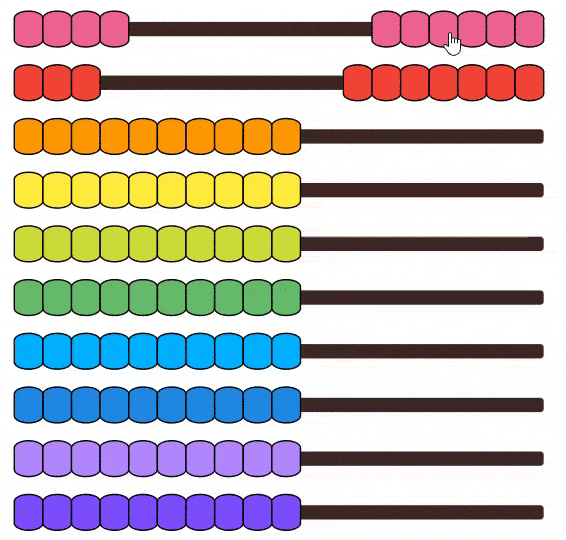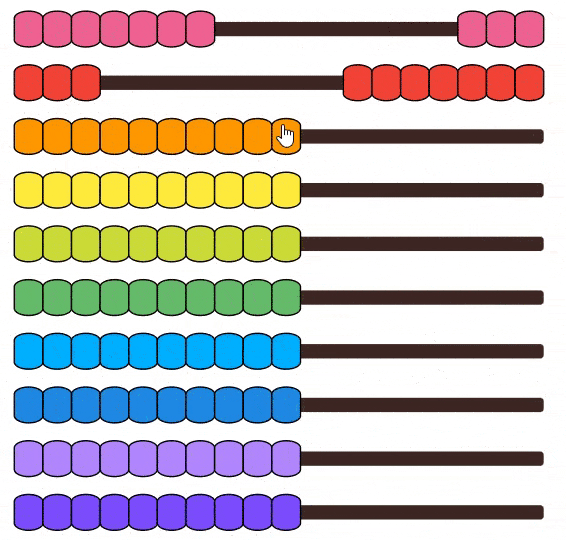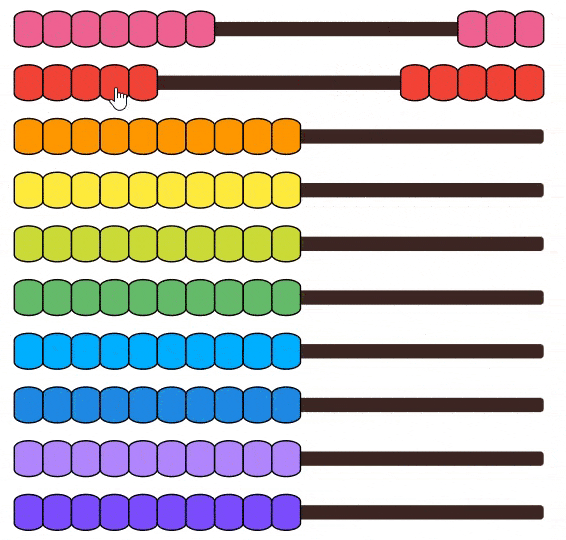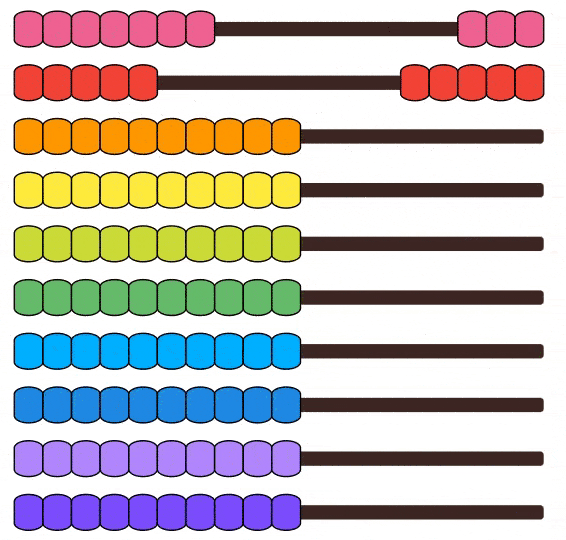
Double Digit Subtraction on Abacus Step-by-Step Process
First, begin by representing 67 on the abacus: 6 beads on the tens' row and 7 beads on the ones' row.
Next, we need to minus 32. As 3 is on tens place, so push aside 3 beads from the tens' row.
In the ones place, we have 2. So, slide 2 beads from from right to left on the ones' row.
Now, count the rest of the beads and you have the answer. Young kids often find it challenging to learn subtraction. If your little one is also struggling with it, you can try the above method method to teach how to subtract double-digit numbers.
Young kids often find it challenging to learn subtraction. If your little one is also struggling with it, you can try the above method method to teach how to subtract double-digit numbers.
Method 3
Subtracting Triple-Digit Numbers on Abacus Try to solve
Try to solve 653-159 on Abacus.
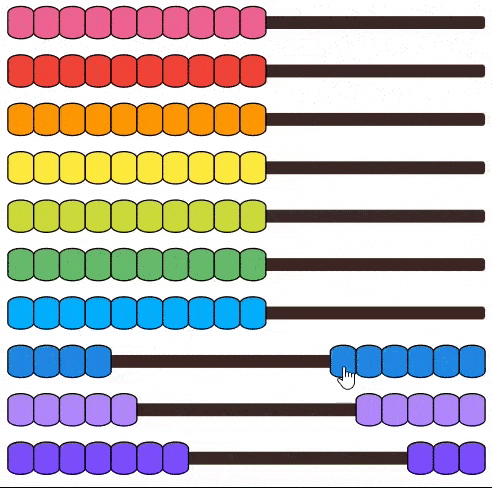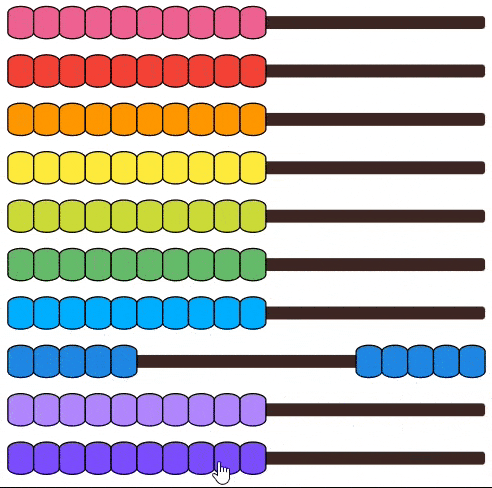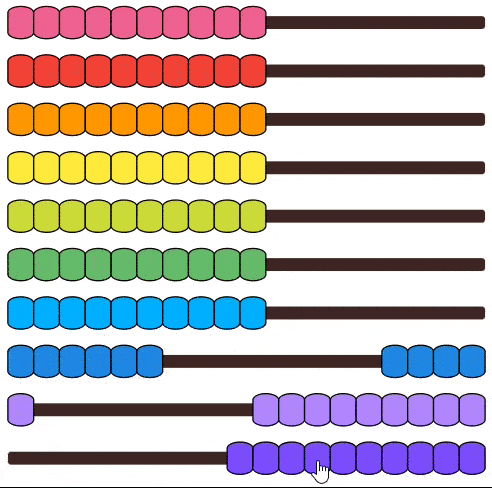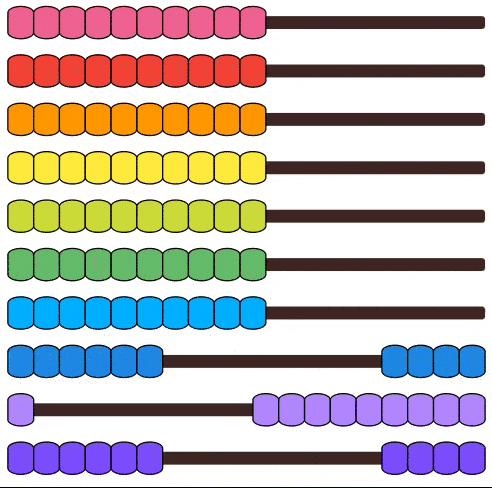
Three Digit Subtraction on Abacus Step-by-Step Process
First, begin by representing 653 on the abacus: 6 beads on the hundredths-row, 5 beads on the tens-row, and 3 beads on the ones-row.
Next, we need to subtract 159. As 1 is on hundredths-row tens place, so push aside 1 bead from right to left on the hundredths-row.
In the tens place, we have 5. So, slide all the 5 beads from from right to left on the tens-row.
Similarly, you need to push 9 beads from right to left on the ones row.
However, after sliding the 3 beads when you're out of beads, you need to trade 1 bead on tens-row for 10 beads on the right on the ones-row.
But, as you can see, there are no beads on the tens-row. So, go to hundredths-row and push 1 bead to the left to trade 10 beads on tens-row.
Now, you can slide 1 bead from right to left on tens-row and bring 10 beads on ones-row.
We need to subtract total 9 beads. As we have already subtracted 3 beads, now, it's time to subtract 6 more beads, leaving behind only 4 beads on the ones-row.
Lastly, you have the answer: 494


6. Multiplication
Understanding Multiplication Using an Abacus once the new learners get accustomed to using the abacus and pushing the beads from one corner to another, I think they are ready to learn how to use an abacus to multiply.
But one cautionary tip: don’t try to teach multiplication before they learn how to add and subtract. For this mathematical operation, let's try to understand how 3×4=12
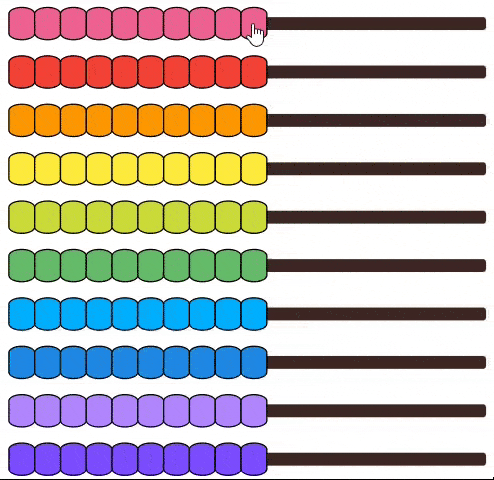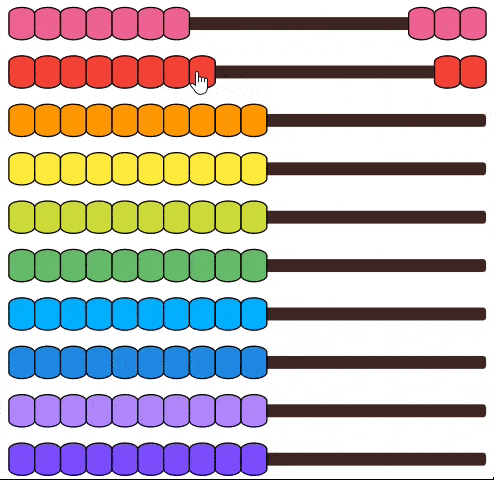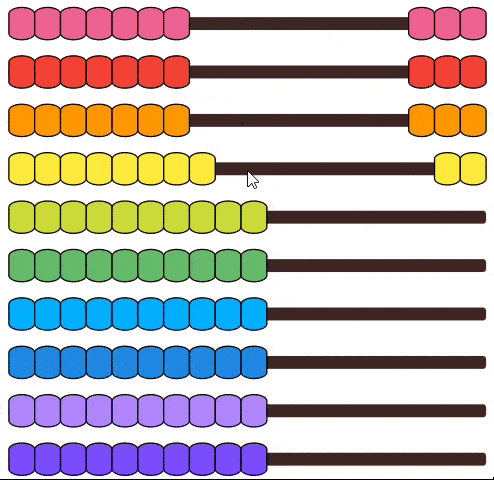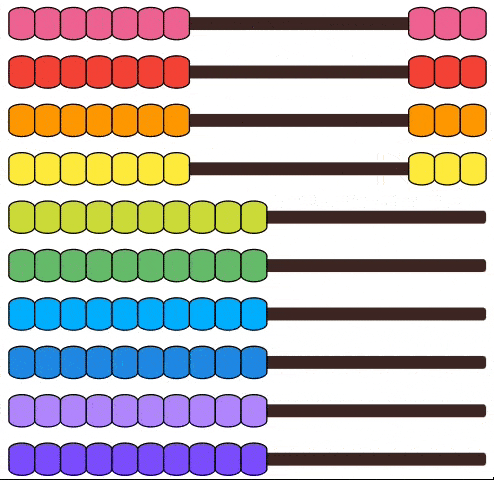
Explain Multiplication on Abacus Ask your kid to arrange 3 beads on 4 different rows and then ask them to count. Through this process, they will have a mental picture of how 4 times 3 makes a 12.
Remember, this process is only to understand how multiplication works.
Method 1
Multiplying Single Digit Numbers on an Abacus To see how to do multiplication on abacus, let’s look at an example: 6 × 5
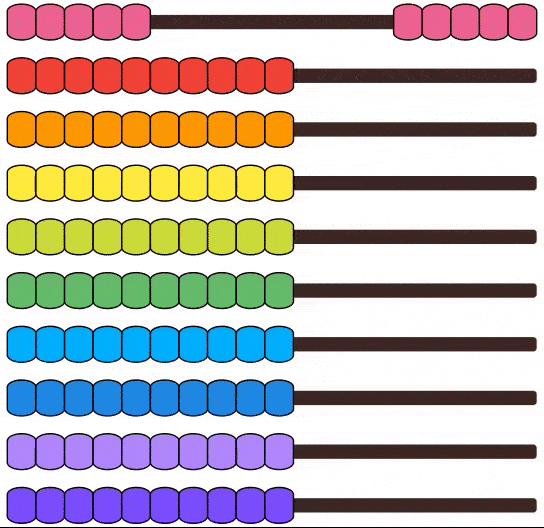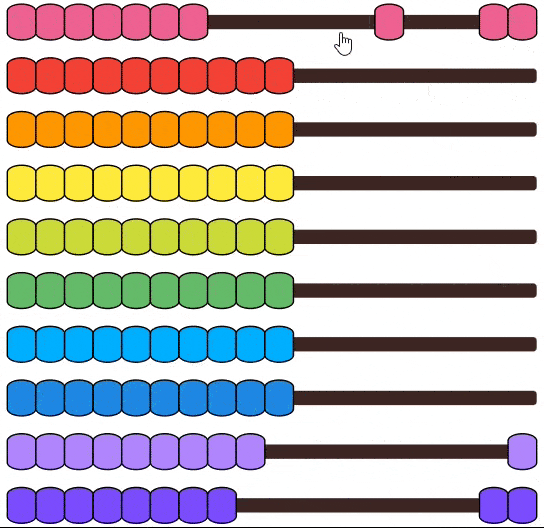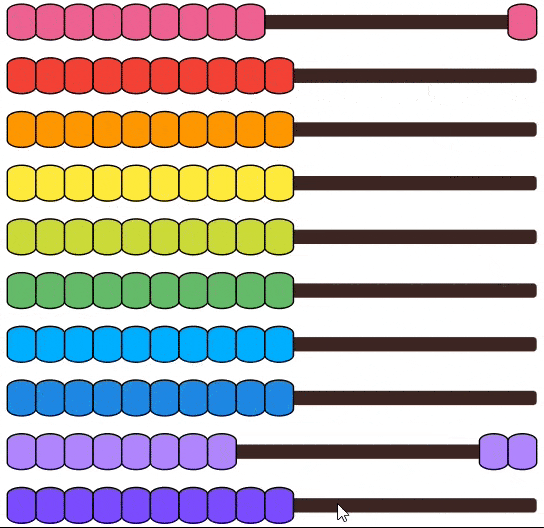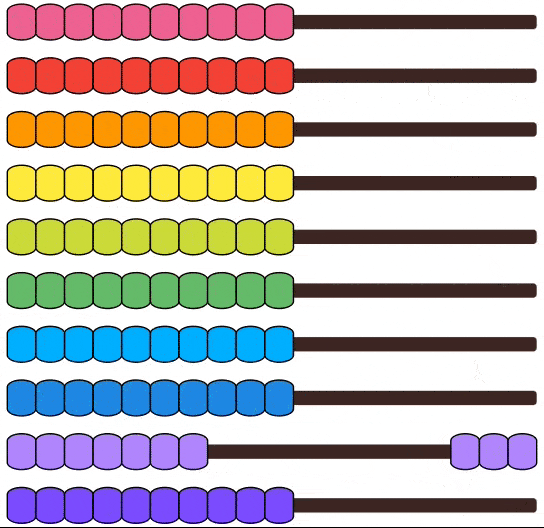
Multiplying Single Digit Numbers on Abacus For this, the learner must know how to represent place values.
So, let’s begin by considering the last row and the first row as the ones. Why do we need two rows? Because you are multiplying.
Step-by-Step Process
Begin by representing 5 on the first row with 5 beads on the right.
Move 1 bead (from the 5 beads) on the top row to the left, then, slide 6 beads on the bottom row to the right.
Again, move another bead towards the left. This time you need to add 6 more beads on the bottom row.
So, you keep sliding…1…2…3…4 beads till you run out of beads.
Now, trade all the beads on the ones-row (slide to the left) for1 bead on the tens-row towards the right.
After that, keep adding beads on the ones-row towards the right and count…5…6.
Continue by pushing the 3rd bead from the top row towards the left and add another 6 beads on the bottom row.
Keep repeating the steps until you have 0 beads on the top row and bottom row and 3 beads on the tens row.
Now, you can already guess the answer: 3 tens equals 30.

Multiplying Double Digit Number on an Abacus If you want to
If you want to multiply double digit numbers, such as 21×5, you need to take two rows from the top as ones and tens, just like you did for the bottom rows in the last example.
Always represent the bigger number in the bottom. Why? It’s convenient.
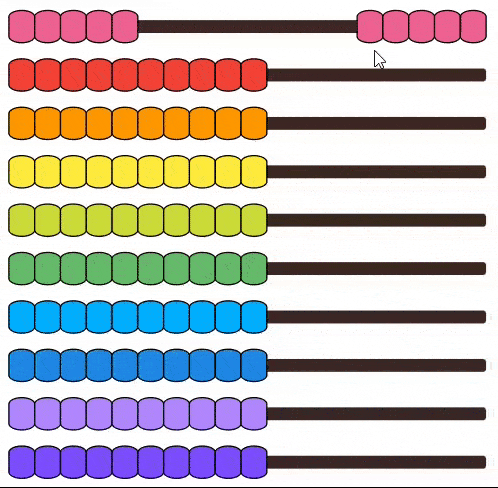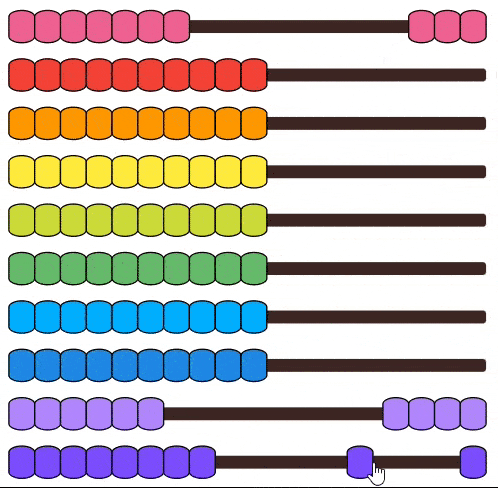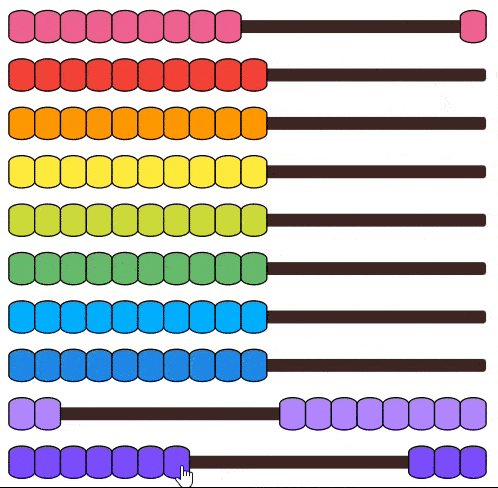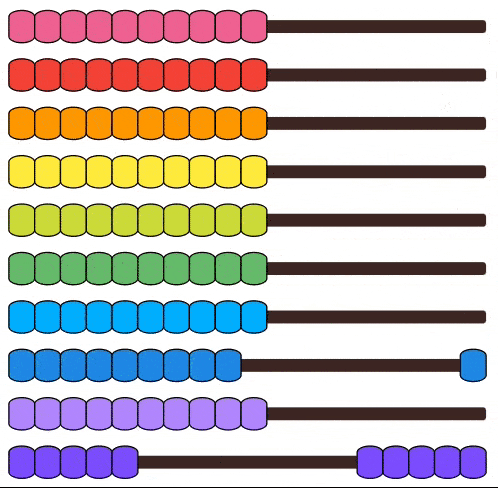
Multiplying Double Digit on Abacus gif Step-by-Step Process
Arrange 5 beads on the top row (consider it as ones row).
Next, take 2 beads on the right side of the bottom tens row and 1 bead on the ones row to represent 21.
Now, each time you slide 1 bead from the top “ones row” towards the left, you will need to add another 21. Keep repeating this until you are out of tens-beads.
When you are out of beads on the tens-row, you have 1 hundred, so add 1 bead on the “hundred-row” and push all the tens beads towards the left to continue counting afresh.
Keep doing this until you have that 1 bead on the hundred-row, 0 beads on the tens-row, and 5 beads on the ones-row.
The answer is 105

Let's try to multiply 143 × 4.
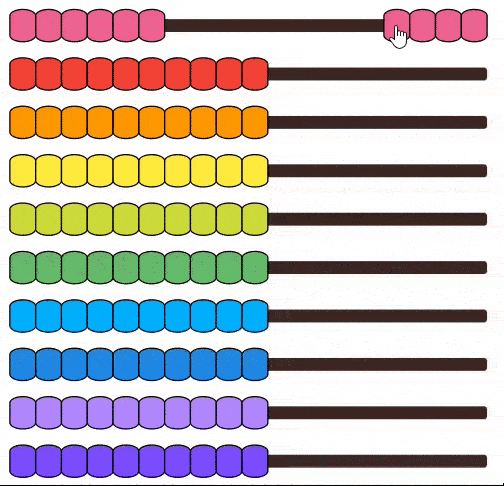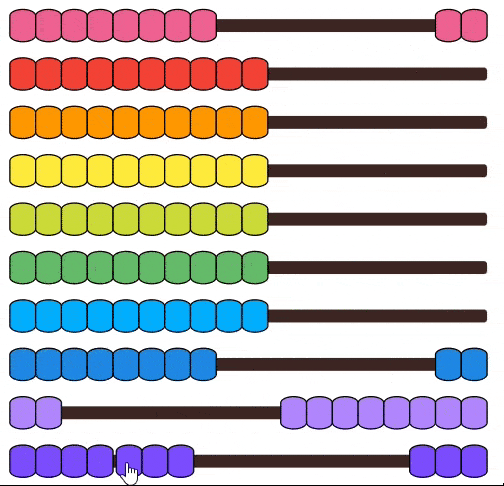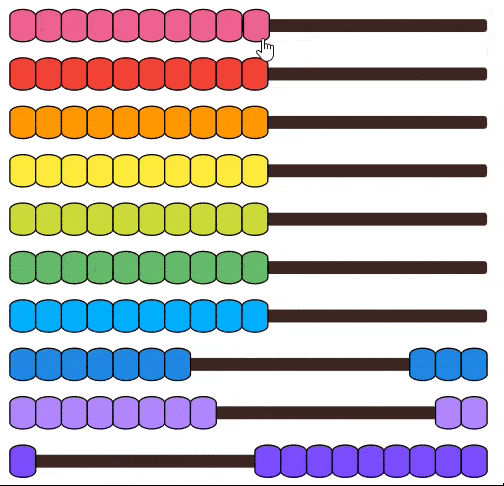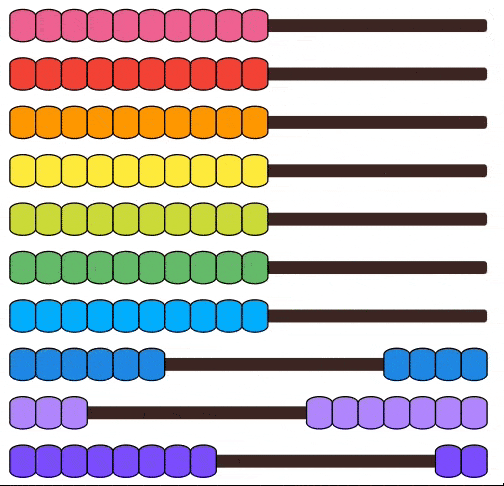
143×4=572 on Abacus Step-by-Step Process
Arrange 4 beads on the top row.
Now, slide all the 4 beads from right to left one by one.
Each time you slide 1 bead to left, you need to add 143 on the bottom rows.
How to add 143 on the abacus? Slide 1 bead on the hundredth-row (from the bottom), 4 beads on the tens row, and 3 beads on the ones-row to represent 143.
Now, each time you slide 1 bead from the top “ones row” to left, add another 143. Keep repeating this until you are out of tens-beads.
When you are out of beads on the tens-row, you have 1 hundred, so add 1 bead on the “hundredth-row” and push all the tens beads towards the left to continue counting afresh.
Keep doing this until you have that 5 beads on the hundredth-row, 7 beads on the tens-row, and 2 beads on the ones-row.
The answer is 572.
7. Division
Understanding Division on an Abacus The division is one of the basic four math operations, and it's recommended to teach after the students know the former ones.
Therefore, before teaching division on an abacus, please make sure your kid knows how to add, subtract, and multiply on the abacus.
Go through the concepts of dividend and divisor. Always represent the dividend on the top rows and the divisor on the bottom rows.
For division operations, explain which rows are ones, tens, hundreds, and so on. First rows from the top and bottom: ones-row Second rows from the top and bottom: tens-row Third rows from the top and bottom: hundredth-row
Method 1
Dividing A Single Digit Number on an Abacus Always begin with an easy example for kids. Let's take 9÷3. For division, represent the dividend on the top row.
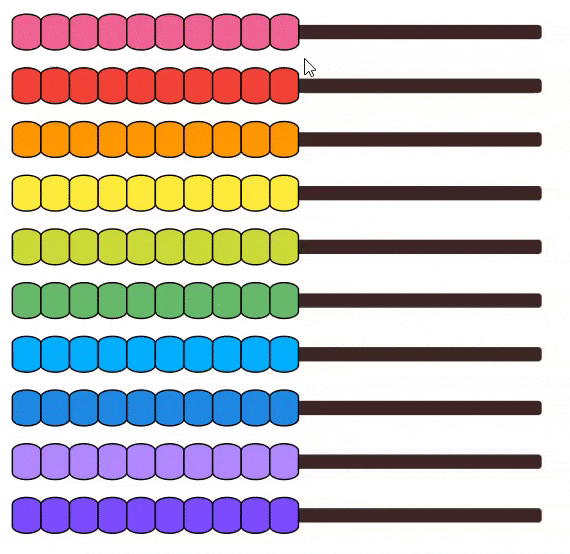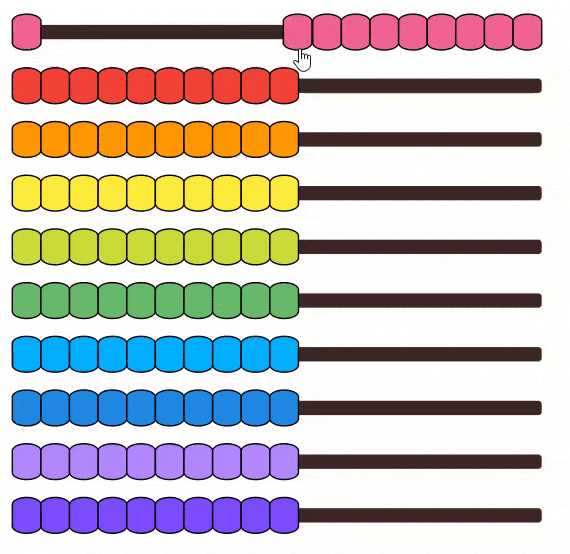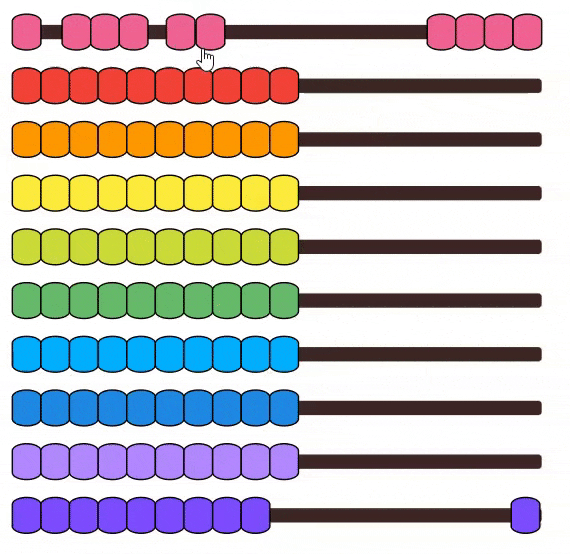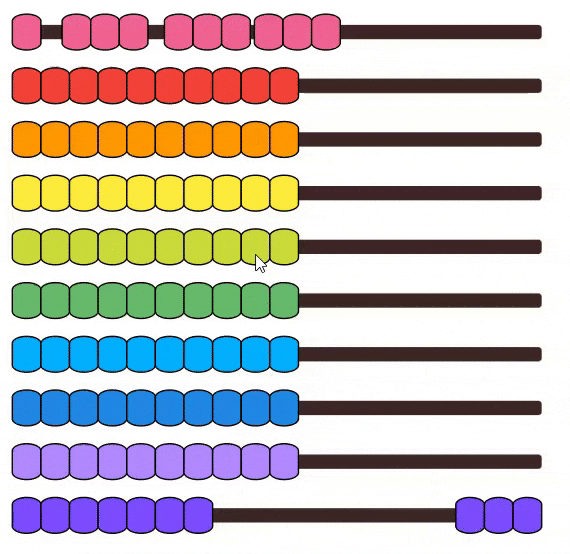
Step-by-Step Process
Slide 9 beads towards the right on the top row, as shown in the above image.
Next, as you know, the divisor is 3, so keep sliding 3 beads at a time towards the left on the top row.
For each set of 3 beads, slide 1 bead towards the right on the bottom row.
At the end of the operation (as shown on the image), you will have 3 beads on the bottom row.
Therefore, the quotient is 3 and 0 beads on the right side of the top row, so 0 is the remainder.
Method 2
How to Divide A Double Digit Number on an Abacus
Example 1 Let's try to divide 10 ÷ 4

Step-by-Step Process
Represent the dividend by sliding 10 beads towards the right on the top.
The divisor is 4.
So, keep moving 4 beads at a time from right to left on the first row.
For each set of 4-beads, slide 1 bead towards the right on the bottom row. '
In the end, you will have 2 beads, both on the right side of the bottom row and on the top row.
Therefore, the quotient is 2, and the remainder is also 2.
Example 2 Let's divide 25÷5
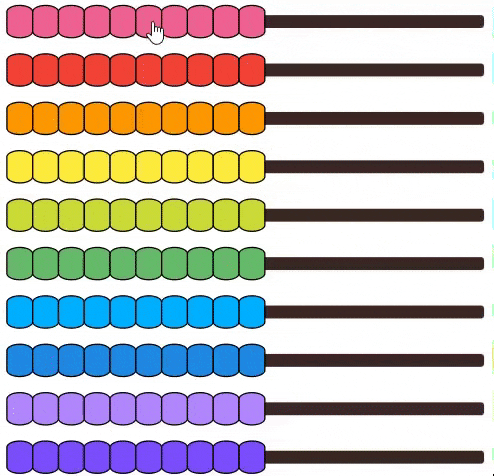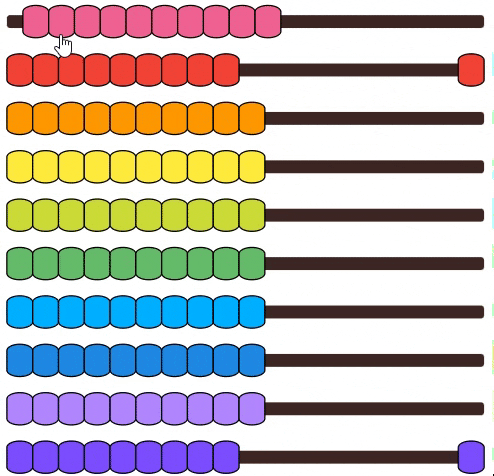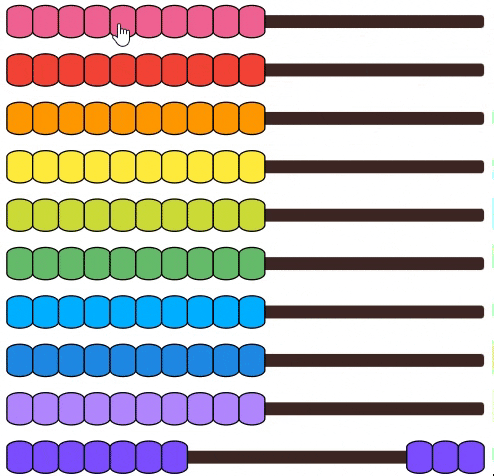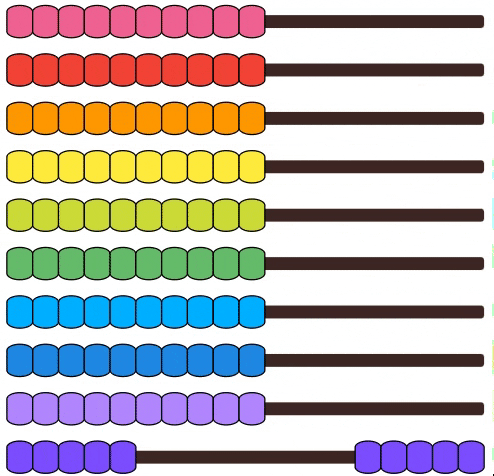
Like you did in the multiplication examples, you need to take two rows from the top to represent a double-digit number other than 10. As they already know, 25 has 2 tens and 5 ones. Step-by-Step Process
Slide 2 beads and 5 beads to the right on the second and first rows from the top, respectively. There you have 25.
Now, as the divisor is 5, keep sliding 5 beads on the top row, and for each set of 5 beads, you push 1 bead towards the right on the bottom row.
When you have 1 bead on the bottom, you will run out of beads on the top row.
So, what to do?
Next, you slide a bead from the tens-row towards the left and bring 10 beads on the ones-row in exchange for that.
Now you have enough beads for another 2 beads on the bottom row. Keep going like this until you have 5 beads on the bottom row.
The quotient is 5, and the remainder is 0.
Method 3
How to Divide a Three-Digit Number Using an Abacus Let's try to divide 225 ÷ 25
Step-by-Step Process
To represent the dividend 225, slide 2 beads on the hundreds-row, 2 beads on the tens-row, and 5 beads on the ones-row in the top.
Now, like the previous example, keep reducing 25 (2 tens-row beads and 5 ones-row beads) from left to right on the top.
For each set of 25, slide 1 bead towards the left on the bottom ones-row.
After pushing the first set of 25, the top tens-row is empty.
So, you slide 1 bead on the hundreds-row from left to right and, in exchange, get 10 beads on the tens-row.
Now, keep sliding 2 tens-row beads and 5 ones-row beads till you get 9 beads on the bottom ones-row.
The quotient is 9, and the remainder is 0.
This is how you divide on an abacus. If you still have any confusion regarding the sums on abacus, please watch this video.
Things to Keep in Mind While Teaching Abacus to Kids
Give a Proper Introduction to the Abacus Kids
Who use the counting device after joining the school find using the abacus very overwhelming. If you want your little one not to panic and be comfortable with the counting frame, then please introduce the device properly.
First, teach them how to make the beads read 5, 6, or other numbers. Teach them how to count and how to operate the beads.
Teach Kids How to Represent Bigger Numbers
To do Math on Abacus You should at least teach kids how to represent three-digit numbers on the Danish abacus before diving into basic operations.
Always Begin with One-Digit Operations
In the beginning, everybody finds the ancient calculator odd. Therefore, I suggest every parent should teach how to add / subtract / multiply / divide single-digit numbers before dealing with bigger numbers.
Be Patient
It will take a significant time before your kid begins to solve math problems on the abacus. So, get ready to be patient. Hang in there with your child. Give me confidence.
Encourage them. Remember, if you get frustrated, they will give up way sooner than you expect.
Turn the Math Problems Into Game As a parent
you might have already observed how kids react when any chore is fun and happening. If you're struggling to capture your child's attention, then try turning the abacus-related problems into games.
Give them points after every successful operation. To make it even more interesting, you can also add rewards.
Buy Abaci with Colorful
Beads The colors are catchy. Kids prefer abaci with colorful beads to the ones in dull colors. So, always try to pick a device that your kids are going to like. You can also ask their opinion before buying it. Now, it's time for a quick abacus quiz.
Quiz
Test What You Have Learnt Dear readers, grab your abacus and try to find answer to the following sums.
A friendly caution of warning: Don't use the modern calculator. Let your fingers find the answer on the beads. [ays _ quiz id = "5"]


Conclusion
Undoubtedly, an abacus is the most efficient tool for conducting basic math operations. You may be a teacher or a parent; it doesn't matter. If you're struggling with explaining basic math to kids, I suggest you try abacus.
I hope this write-up helps you figure out how to use a simple abacus. Please let me know how your adventure abacus is turning out in the comments.
FAQs
1) What operations can you do on an abacus?
You can do addition, subtraction, multiplication, and division using an abacus. In addition to that, you can also represent bigger numbers on an abacus. Also, one can extract square roots and cube roots on the counting frame.
2) Is an abacus useful for teaching math?
Yes, an abacus is useful for teaching math, especially to preschoolers, kindergartens, and visually impaired learners.
3) What is butterfly number in abacus?
Decimal numbers are referred as butterfly number in abacus because they contain a string of beads while counting.
How do I use an abacus and do mental math on it super fast?
First of all, you don't do mental math on abacus. You learn abacus, practice to calculate fast, and then mentally picturize the abacus in your mind. The mental math process involves imagining the abacus in your mind and moving the beads to calculate without using any physical device.



.png)

Comments