Mathematics is often considered a language, and within its vast landscape, multiplication tables are foundational building blocks. They help children understand other concepts, such as fractions, percentages, and even shapes. Memorizing times tables makes it far quicker and easier for children to work out math problems in their heads. It lays the foundation for algebra, opens the door to multi-digit multiplication, including long multiplication, and demystifies processes like long division and simplifying fractions. In this blog post, we'll explore the concept of multiplication tables, their significance, and some fun tricks to make learning them a breeze.
What are Multiplication Tables?
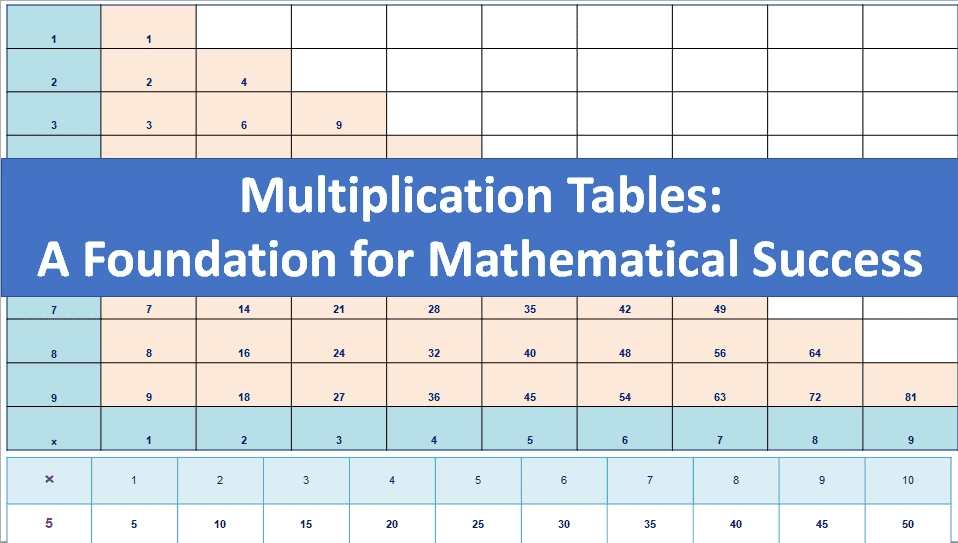
Multiplication tables, or times tables, are a systematic arrangement of numbers representing the result of multiplying two integers. Typically, these tables are presented in a grid format, where each cell contains the product of a particular number from the corresponding row and column. The most common multiplication chart is the 10x10 grid, representing multiplication facts from 1 to 10 for good practice. Math worksheets are often used to practice multiplication facts, including the complete set of questions exactly once on each page, known as a worksheet in conjunction. A 2x multiplication table would show all the products when 2 is multiplied by each integer from 1 to 10—
2 | x | 1 | = | 2 |
2 | x | 2 | = | 4 |
2 | x | 3 | = | 6 |
2 | x | 4 | = | 8 |
2 | x | 5 | = | 10 |
2 | x | 6 | = | 12 |
2 | x | 7 | = | 14 |
2 | x | 8 | = | 16 |
2 | x | 9 | = | 18 |
2 | x | 10 | = | 20 |
The Math Behind Multiplication Tables Multiplication tables are a fundamental concept in arithmetic, representing the result of multiplying two numbers together. Let's break down the math behind multiplication tables with examples—
Basic Multiplication The multiplication of two numbers, a and b, is denoted as a×b or ab, and it is the sum of a added to itself b times.
Multiplication: A mathematical operation that combines two numbers to produce a result called the product.
Notation: It's written as "a × b" or "ab," where a and b are the numbers being multiplied.
Repeated Addition: The essence of multiplication is repeated addition.
Examples:
4 × 3: This means "4 multiplied by 3." It's the same as adding 4 to itself 3 times:4 + 4 + 4 = 12. So,4 × 3 = 12
7 × 2: This means "7 multiplied by 2." It's the same as adding 7 to itself 2 times:7 + 7 = 14. So,7 × 2 = 14.
Multiplication Table A multiplication table is a grid that shows the products of all pairs of numbers within a certain range. The most common multiplication table is the times table up to 10. Let's take the example of the 5 times table:
× | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
5 | 5 | 10 | 15 | 20 | 25 | 30 | 35 | 40 | 45 | 50 Each entry in the table is the product of the corresponding row number and column number. For instance, in the |
Each entry in the table is the product of the corresponding row number and column number. For instance, in the 5 times table, the entry in the row labeled 5 and the column labeled 4 is 5×4=20.
Patterns in Multiplication Tables:-
Diagonal Pattern
The diagonal of a multiplication table (from the top left to the bottom right) contains the square of the numbers.
Example: In the 5 times table, the diagonal elements are12, 22, 32, …,102.
1 | 1 |
|
|
|
|
|
|
|
|
2 | 2 | 4 |
|
|
|
|
|
|
|
3 | 3 | 6 | 9 |
|
|
|
|
|
|
4 | 4 | 8 | 12 | 16 |
|
|
|
|
|
5 | 5 | 10 | 15 | 20 | 25 |
|
|
|
|
6 | 6 | 12 | 18 | 24 | 30 | 36 |
|
|
|
7 | 7 | 14 | 21 | 28 | 35 | 42 | 49 |
|
|
8 | 8 | 16 | 24 | 32 | 40 | 48 | 56 | 64 |
|
9 | 9 | 18 | 27 | 36 | 45 | 54 | 63 | 72 | 81 |
× | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
Commutative Property Multiplication is commutative, meaning a×b=b×a. This property is reflected in the symmetry of the multiplication table across its main diagonal. Example:
5 x 3 = 15 (5 groups of 3)
3 x 5 = 15 (3 groups of 5)
The product is the same (15) in both cases.
Notice how 5 x 3 = 15 and 3 x 5 = 15 are mirror images in the table.
1 | 2 | 3 | 4 | 5 |
2 | 4 | 6 | 8 | 10 |
3 | 6 | 9 | 12 | 15 |
4 | 8 | 12 | 16 | 20 |
5 | 10 | 15 | 20 | 25 |
Multiples Each row or column represents the multiples of the corresponding number. In the 5 times table, the fifth row represents multiples of 5, and the fifth column also represents multiples of 5.
Example: Suppose you want to find the total number of fingers on 8 people. You can use the multiplication table for 8: 8×1=8 8×2=16 8×3=24 ⋮ 8×10=80 Adding up these values gives the total: 8+16+24+…+80 Multiplication Tables (1 to 5) Free Printable times table charts and tables are provided to help you learn times tables effortlessly. These times tables charts help the students to memorize them faster if they frequently revise them and take a speed test.
1 x 1 = 1 | 2 x 1 =2 | 3 x 1 = 3 | 4 x 1 =4 | 5 x 1 =5 |
1 x 2 = 2 | 2 x 2 = 4 | 3 x 2 = 6 | 4 x 2 =8 | 5 x 2 =10 |
1 x 3 = 3 | 2 x 3 = 6 | 3 x 3 = 9 | 4 x 3 =12 | 5 x 3 =15 |
1 x 4 = 4 | 2 x 4 = 8 | 3 x 4 = 12 | 4 x 4 =16 | 5 x 4 =20 |
1 x 5 = 5 | 2 x 5 = 10 | 3 x 5 = 15 | 4 x 5 =20 | 5 x 5 =25 |
1 x 6 = 6 | 2 x 6 = 12 | 3 x 6 = 18 | 4 x 6 = 24 | 5 x 6 =30 |
1 x 7 = 7 | 2 x 7 = 14 | 3 x 7 = 21 | 4 x 7 = 28 | 5 x 7 = 35 |
1 x 8 = 8 | 2 x 8 = 16 | 3 x 8 = 24 | 4 x 8 = 32 | 5 x 8 =40 |
1 x 9 =9 | 2 x 9 =18 | 3 x 9 = 27 | 4 x 9 = 36 | 5 x 9 =45 |
1 x 10 =10 | 2 x 10 =20 | 3 x 10 =30 | 4 x 10 =40 | 5 x 10 =50 |
Multiplication Tables (5 to 10)
6 x 1 =6 | 7 x 1 =7 | 8 x 1 =8 | 9 x 1 =9 | 10 x 1 =10 |
6 x 2 =12 | 7 x 2 =14 | 8 x 2 =16 | 9 x 2 =18 | 10 x 2 =20 |
6 x 3 =18 | 7 x 3 =21 | 8 x 3 = 24 | 9 x 3 = 27 | 10 x 3 =30 |
6 x 4 =24 | 7 x 4 =28 | 8 x 4 =32 | 9 x 4 =36 | 10 x 4 =40 |
6 x 5 =30 | 7 x 5 =35 | 8 x 5 =40 | 9 x 5 =45 | 10 x 5 =50 |
6 x 6 =36 | 7 x 6 =42 | 8 x 6 =48 | 9 x 6 =54 | 10 x 6 = 60 |
6 x 7 =42 | 7 x 7 =49 | 8 x 7 = 56 | 9 x 7 = 63 | 10 x 7 = 70 |
6 x 8 =48 | 7 x 8 =56 | 8 x 8 =64 | 9 x 8 = 72 | 10 x 8 =80 |
6 x 9 = 54 | 7 x 9 = 63 | 8 x 9 = 72 | 9 x 9 =81 | 10 x 9 =90 |
6 x 10 = 60 | 7 x 10 = 70 | 8 x 10 = 80 | 9 x 10 =90 | 10 x 10 =100 |
Multiplication Tables (11 to 20)
11 x 1 = 11 | 12 x 1 = 12 | 13 x 1 = 13 | 14 x 1 = 14 | 15 x 1 = 15 | 16 × 1 = 16 | 17 × 1 = 17 | 18 × 1 = 18 | 19 × 1 = 19 | 20 × 1 = 20 |
11 x 2 = 22 | 12 x 2 = 24 | 13 x 2 = 26 | 14 x 2 = 28 | 15 x 2 = 30 | 16 × 2 = 32 | 17 × 2 = 34 | 18 × 2 = 36 | 19 × 2 = 38 | 20 × 2 = 40 |
11 x 3 = 33 | 12 x 3 = 36 | 13 x 3 = 39 | 14 x 3 = 42 | 15 x 3 = 45 | 16 × 3 = 48 | 17 × 3 = 51 | 18 × 3 = 54 | 19 × 3 = 57 | 20 × 3 = 60 |
11 x 4 = 44 | 12 x 4 = 48 | 13 x 4 = 52 | 14 x 4 = 56 | 15 x 4 = 60 | 16 × 4 = 64 | 17 × 4 = 68 | 18 × 4 = 72 | 19 × 4 = 76 | 20 × 4 = 80 |
11 x 5 = 55 | 12 x 5 = 60 | 13 x 5 = 65 | 14 x 5 = 70 | 15 x 5 = 75 | 16 × 5 = 80 | 17 × 5 = 85 | 18 × 5 = 90 | 19 × 5 = 95 | 20 × 5 = 100 |
11 x 6 = 66 | 12 x 6 = 72 | 13 x 6 = 78 | 14 x 6 = 84 | 15 x 6 = 90 | 16 × 6 = 96 | 17 × 6 = 102 | 18 × 6 = 108 | 19 × 6 = 114 | 20 × 6 = 120 |
11 x 7 = 77 | 12 x 7 = 84 | 13 x 7 = 91 | 14 x 7 = 98 | 15 x 7 = 105 | 16 × 7 = 112 | 17 × 7 = 119 | 18 × 7 = 126 | 19 × 7 = 133 | 20 × 7 = 140 |
11 x 8 = 88 | 12 x 8 = 96 | 13 x 8 = 104 | 14 x 8 = 112 | 15 x 8 = 120 | 16 × 8 = 128 | 17 × 8 = 136 | 18 × 8 = 144 | 19 × 8 = 152 | 20 × 8 = 160 |
11 x 9 = 99 | 12 x 9 = 108 | 13 x 9 = 117 | 14 x 9 = 126 | 15 x 9 = 135 | 16 × 9 = 144 | 17 × 9 = 153 | 18 × 9 = 162 | 19 × 9 = 171 | 20 × 9 = 180 |
11 x 10 = 110 | 12 x 10 = 120 | 13 x 10 = 130 | 14 x 10 = 140 | 15 x 10 = 150 | 16 × 10 = 160 | 17 × 10 = 170 | 18 × 10 = 180 | 19 × 10 = 190 | 20 × 10 = 200 |
Multiplication Tables (21 to 30)
21 × 1 = 21 | 22 × 1 = 22 | 23 × 1 = 23 | 24 × 1 = 24 | 25 × 1 = 25 | 26 x 1 = 26 | 27 x 1 = 27 | 28 x 1 = 28 | 29 x 1 = 29 | 30 x 1 = 30 |
21× 2 = 42 | 22 × 2 = 44 | 23 × 2 = 46 | 24 × 2 = 48 | 25 × 2 = 50 | 26 x 2 = 52 | 27 x 2 = 54 | 28 x 2 = 56 | 29 x 2 = 58 | 30 x 2 = 60 |
21 × 3 = 63 | 22 × 3 = 66 | 23 × 3 = 69 | 24 × 3 = 72 | 25 × 3 = 75 | 26 x 3 = 78 | 27 x 3 = 81 | 28 x 3 = 84 | 29 x 3 = 87 | 30 x 3 = 90 |
21 × 4 = 84 | 22 × 4 = 88 | 23 × 4 = 92 | 24 × 4 = 96 | 25 × 4 = 100 | 26 x 4 = 104 | 27 x 4 = 108 | 28 x 4 = 112 | 29 x 4 = 116 | 30 x 4 = 120 |
21 × 5 = 105 | 22 × 5 = 110 | 23 × 5 = 115 | 24 × 5 = 120 | 25 × 5 = 125 | 26 x 5 = 130 | 27 x 5 = 135 | 28 x 5 = 140 | 29 x 5 = 145 | 30 x 5 = 150 |
21 × 6 = 126 | 22 × 6 = 132 | 23 × 6 = 138 | 24 × 6 = 144 | 25 × 6 = 150 | 26 x 6 = 156 | 27 x 6 = 162 | 28 x 6 = 168 | 29 x 6 = 174 | 30 x 6 = 180 |
21 × 7 = 147 | 22 × 7 = 154 | 23 × 7 = 161 | 24 × 7 = 168 | 25 × 7 = 175 | 26 x 7 = 182 | 27 x 7 = 189 | 28 x 7 = 196 | 29 x 7 = 203 | 30 x 7 = 210 |
21 × 8 = 168 | 22 × 8 = 176 | 23 × 8 = 184 | 24 × 8 = 192 | 25 × 8 = 200 | 26 x 8 = 208 | 27 x 8 = 216 | 28 x 8 = 224 | 29 x 8 = 232 | 30 x 8 = 240 |
21 × 9 = 189 | 22 × 9 = 198 | 23 × 9 = 207 | 24 × 9 = 216 | 25 × 9 = 225 | 26 x 9 = 234 | 27 x 9 = 243 | 28 x 9 = 252 | 29 x 9 = 261 | 30 x 9 = 270 |
21× 10 = 210 | 22 × 10 = 220 | 23 × 10 = 230 | 24 × 10 = 240 | 25 × 10 = 250 | 26 x 10 = 260 | 27 x 10 = 270 | 28 x 10 = 280 | 29 x 10 = 290 | 30 x 10 = 300 |
Creating Multiplication Tables Creating your own multiplication tables can be an engaging and effective learning method. While most multiplication tables are already created and available in textbooks or online, you can also construct them on yourselves.
Start with a blank grid and fill in each cell by multiplying the corresponding row and column numbers.
Color-coding or using distinct symbols for even and odd numbers can enhance visual memory.
Across the top row and left column, write out the numbers 1 through 10.
Then fill out each box by multiplying the top number by the left number, writing the product in the box. For example, the box for 6 x 4 would show 24.
Fill out the entire table through 10 x 10.
1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | |
1 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
2 | 2 | 4 | 6 | 8 | 10 | 12 | 14 | 16 | 18 | 20 |
3 | 3 | 6 | 9 | 12 | 15 | 18 | 21 | 24 | 27 | 30 |
4 | 4 | 8 | 12 | 16 | 20 | 24 | 28 | 32 | 36 | 40 |
5 | 5 | 10 | 15 | 20 | 25 | 30 | 35 | 40 | 45 | 50 |
6 | 6 | 12 | 18 | 24 | 30 | 36 | 42 | 48 | 54 | 60 |
7 | 7 | 14 | 21 | 28 | 35 | 42 | 49 | 56 | 63 | 70 |
8 | 8 | 16 | 24 | 32 | 40 | 48 | 56 | 64 | 72 | 80 |
9 | 9 | 18 | 27 | 36 | 45 | 54 | 63 | 72 | 81 | 90 |
10 | 10 | 20 | 30 | 40 | 50 | 60 | 70 | 80 | 90 | 100 |
The Importance of Multiplication Tables Mastering multiplication tables is crucial for building a solid foundation in mathematics. These tables serve as a gateway to more advanced concepts, including division, fractions, and algebra. Having quick access to these multiplication facts, also known as multiplication tables, is hugely beneficial across math disciplines like fractions, ratios, proportions, division, algebra, and more. Multiplication tables at primary school transform longer computations into rapid mental reflexes and are helpful in quick calculations. Building fluency takes practice through repetition, but it saves much time and effort down the road. Early mastery of times tables at primary school gives students a useful tool to apply to all higher math studies in conjunction. A strong grasp of multiplication lays the groundwork for efficient mental math and problem-solving skills in everyday life.
Interesting Facts and Concepts
Commutative Property: Multiplication tables exhibit the commutative property, meaning that the order of numbers doesn't affect the product. For example,2×3 is the same as3×2.
Zero Property: Any number multiplied by zero is always zero. This fundamental concept is reflected in the multiplication tables.
Double-Trouble: Doubling a number is the same as multiplying by 2. So,8 x 5 is the same as doubling 5 twice: 5 x 2 = 10, then 10 x 2 = 20.
5 & 6 Combo: Multiplying by 6 is like multiplying by 5 and adding the original number. For example, 6 x 4 = (5 x 4) + 4 =20 + 4 =24.
Patterns and Symmetry: Multiplication tables often showcase intriguing patterns and symmetries. Identifying and understanding these patterns can aid in quick mental calculations.
9 is frequently considered a “tricky” number, but any number multiplied by 9 has a cool pattern - simply hold up your hands and the number of fingers to the left of the raised fingers is the tens digit while the number of fingers to the right is the ones digit. For example, 7 fingers to the left of 2 raised fingers means 7 x 9 = 63!
Multiplying by 5 is easy since the products always end in a 0 or 5.
The squares of numbers follow interesting patterns. For all integers, if the tens digit is an odd number, the ones digit of its square will be 1. If the tens digit is even, the ones digit of its square will be 4.
How to master Multiplication Tables? Learning and mastering basic multiplication doesn't have to be boring! Approach it like a game with tricks, puzzles, and satisfying “aha!” moments.
Start with the easiest table like the 2s and 5s. Once you know those well, build up to trickier ones.
Create flashcards with the facts you need extra practice on. Drill yourself with quickfire quizzes.
Recite tables out loud to the rhythm of a song or rhyme. This audio/verbal reinforcement helps solidify the facts.
Take practice quizzes and times tests to check your progress. Identify which products you still need more drilling on.
Looking for math instructor? Hire EnthuZiastic Math Experts to master in math no time.
FAQs 1. What is the fundamental concept behind multiplication tables? The fundamental concept behind multiplication tables is the idea that multiplication is a form of repeated addition. Each cell in the table represents the product of multiplying the corresponding row and column numbers. 2. How can you make the process of memorizing multiplication tables more enjoyable? To make memorization enjoyable, one can use methods like flashcards, games, and mnemonic devices. Associating numbers with memorable images or phrases can enhance the learning experience. 3. Why are multiplication tables considered crucial for building a foundation in mathematics? Mastering multiplication tables is essential because they serve as a foundation for more advanced mathematical concepts such as division, fractions, and algebra. A strong grasp of multiplication is key to developing efficient mental math and problem-solving skills. 4. Why is it important to learn multiplication tables? Learning multiplication tables is important because it forms the foundation for more complex mathematical concepts and calculations. By memorizing these tables, students can quickly and accurately perform calculations, develop problem-solving skills, and gain confidence in their mathematical abilities. 5. What are some effective strategies for memorizing multiplication tables? Some effective strategies for memorizing multiplication tables include using flashcards, practicing regularly, breaking down the tables into smaller chunks, using mnemonic devices or patterns, and incorporating fun games and activities to make learning more engaging and enjoyable.
