Odd Numbers From 1 to 1000 

"Mathematics is an universal language. And numbers are its soul." Numbers show various properties according to situations. They range from prime numbers to rational numbers.

Characteristics of Numbers in Mathematics.

These categories provide a framework for understanding the diverse properties and characteristics of numbers in mathematics. Similarly, odd and even numbers play a surprisingly important role in both mathematics and the real world, though their importance varies depending on the context. Even numbers are integers that are divisible by 2, while odd numbers are integers that are not divisible by 2 and can be represented on a number line. Understanding the concept of odd numbers is essential in developing a strong foundation in mathematics.

1. While Odd and Even Numbers Might Seem Simple

Their impact extends far beyond basic counting. They are fundamental tools in mathematics, play a role in countless real-world applications, and even hold cultural and symbolic significance in some societies. In this blog, we will explore the importance of odd numbers and the fundamentals of their properties.

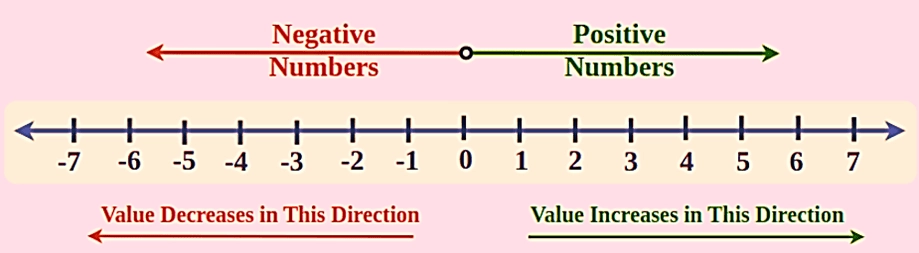
Odd Numbers

Odd numbers are a subset of integers that cannot be divided evenly by 2. In other words, when an odd number is divided by 2, it does not result in a whole number. Instead, there is always a remainder of 1.

Examples:

Odd numbers include 1, 3, 5, 7, 9, and 11.

• 3 ÷ 2 = 1 ( Remainder 1)

• 5 ÷ 2 = 2 ( Remainder 1)

• 7 ÷ 2 = 3 ( Remainder 1)

Identifying Odd Numbers

The best way to spot an odd number is to divide it by 2. If the number does not divide evenly, leaving a remainder of 1, then it is an odd number.

Example:

5/2 = 2 with remainder 1.

7/2 = 3 with remainder 1.

So 5 and 7 are odd numbers.

On the flip side, even numbers can be divided by 2 evenly without leaving any remainder.

Example:

6 / 2 = 3

and

8 / 2 = 4

No remainder!

This is why even numbers end in 0, 2, 4, 6 or 8.

2. Why are Odd Numbers important to learn?

Introducing the concept of even numbers to children after they have a grasp on odd numbers can further enhance their understanding and application of numbers in mathematics. Additionally, it is important to note the significance of composite odd numbers, such as 15 and 21, which are formed by multiplying two smaller positive integers or multiplying the number with one. These numbers may seem simple, but they play a crucial role in understanding the properties of numbers and their relationships in mathematics. In fact, understanding composite odd numbers such as 15 and 21, which are formed by adding 2 to the previous odd number, can help in identifying patterns and solving problems in various mathematical concepts, especially in consecutive odd numbers. Solved examples of odd numbers, such as 3 + 5 = 8 and 7 + 3 = 10, can further illustrate the concept of addition of odd numbers and their resulting even numbers. Additionally, understanding odd composite numbers, such as 15 and 21, which have more than two factors or are not prime, can provide a deeper understanding of the properties of numbers.

3. Significance of the Odd Numbers

Beyond their mathematical definition, odd numbers hold a curious significance across various fields. In mathematics, they hold the key to countless theorems and properties, while in numerology, they symbolize individuality, creativity, and dynamism. Historically, odd numbers have been imbued with spiritual meaning in various cultures, often representing completion or good luck. Even in everyday life, we see their influence: from the three wishes in a fairy tale to the single, burning candle symbolizing hope. While seemingly simple, odd numbers weave a fascinating tapestry of meaning, reminding us that even the smallest difference can hold profound significance.

A chart of odd numbers from 1 to 100

Properties of odd numbers

Divisibility:

An odd number cannot be evenly divided by 2. In other words, dividing an odd number by 2 will result in a quotient that is not an integer (there will be a remainder of 1).

9 ÷ 2 =4 ( Remainder 1)

11 ÷ 2 = 5 ( Remainder 1)

Representation:

Odd numbers are commonly represented as 2k+1, where k is an integer. This representation ensures that when you multiply an odd number by 2 and add 1, you get another odd number. It’s very similar to the general form of an even number which is n=2k.

{2k + 1 | k ∈ Z}

n=2k+1

• This means the set of all odd numbers is the collection of all numbers that can be written as the expression 2k + 1, where k is any integer (Z).

• The number n is an odd number if it can be expressed as 2k+1 where k is just another integer.

Example:

7 → 7 = 2 ( 3 ) + 1

23 → 23 = 2 ( 11 ) + 1

49 → 49 = 2 ( 24 ) + 1

Addition and Subtraction:

Example:

3+5=8

9−5=4

Multiplication:

The product of two odd numbers is always odd.

Example:

3 × 5 =15

7 × 9 = 63

Division:

When an odd number is divided by another odd number, the result can be either odd or fractional.

Example:

9 ÷ 3 = 3 (odd)

15 ÷ 5 = 3 (odd)

• The squares of odd numbers like 1, 9, 25, 49, 81, 121 etc. are again odd numbers. This pattern continues for higher odd number squares too.

• The formula for the sum of the first n odd numbers is: Sum=n2

Looking for math instructor? Hire EnthuZiastic Math Experts to master in math no time.

Interesting Facts

• Ancient Greeks considered odd numbers to be masculine and even numbers to be feminine.

• 99 is the greatest two-digit odd number.

• There are a total of 50 odd numbers between 1 to 100.

• The sum of first 500 odd numbers equals 250,000.

• The sum of all the odd numbers from 1 to 100 is 2500.

• The average or mean of all odd numbers between 1 to 100 is 50.

• Odd numbers have an abundance of primes. More than half of all prime numbers are odd numbers.

• Many prime numbers are odd. Prime numbers are those greater than 1 with no positive divisors other than 1 and themselves. Examples include 3, 5, 7, 11, and so forth.

• There are infinitely many odd numbers. You can always find another odd number by adding 2 to the previous one.

1. Odd Numbers from 101 to 200

2. Odd Numbers from 201 to 300

3. Odd Numbers from 301 to 400

4. Odd Numbers from 401 to 500

5. Odd Numbers from 501 to 600

6. Odd Numbers from 601 to 700

7. Odd Numbers from 701 to 800

8. Odd Numbers from 801 to 900

9. Odd Numbers from 901 to 1000

FAQs

1) What is the defining characteristic of odd numbers?

Odd numbers are integers that cannot be divided evenly by 2, leaving a remainder of 1 when divided by 2.

2) How many odd numbers are there from 1 to 1000?

There are 500 odd numbers from 1 to 1000. Odd numbers are integers that cannot be divided evenly by 2 and have a remainder of 1 when divided by 2. In this range, every other number is odd, so there are 500 odd numbers in total.

3) What is the largest odd number between 1 and 1000?

The largest odd number between 1 and 1000 is 999. Odd numbers are numbers that cannot be divided evenly by 2, leaving a remainder of 1 when divided by 2. Since 1000 is an even number, the largest odd number in this range would be 999.

4) Can an odd number be both a perfect square and a prime number? If so, can you provide an example?

No, an odd number cannot be both a perfect square and a prime number.

Here's why:

• A perfect square is an integer which can be expressed as the product of two identical integers. For example, 4 is a perfect square because 4 = 2 x 2.

• An odd number, by definition, is not divisible by 2. This means that when you prime factorize an odd number, you won't have 2 (or any even number) as a factor. Since a perfect square requires multiplying two identical integers, and one of those integers must always be even (2), it follows that an odd number cannot be a perfect square. Therefore, an odd number cannot be both a perfect square and a prime number.

Since a perfect square requires multiplying two identical integers, and one of those integers must always be even (2), it follows that an odd number cannot be a perfect square. Therefore, an odd number cannot be both a perfect square and a prime number.

5) What property of odd numbers states that their addition always results in an even number?

When you add two odd numbers together, the result is always an even number.

Example:

5 + 5 = 10 6) How can you identify odd numbers from 1 to 1000 using the modulo operator?

You can use the modulo operator (%). If a number divided by 2 leaves a remainder of 1, then it's an odd number.

Example:

7 % 2 equals 1 because 7 divided by 2 leaves a remainder of 1. 7) If you subtract 1 from any odd number, what type of number will you get?

If you subtract 1 from any odd number, you will get an even number.

Example:

5 - 1 = 4 8) If you multiply two odd numbers together, do you always get an even or odd number?

An odd number. The product of any two odd numbers will always be odd because any odd number multiplied by 1 (which is technically multiplying by itself) will remain odd.

Book a Free Consultation

enthu.com

FAQs

1. What are odd numbers?

Odd numbers are integers that cannot be divided evenly by 2. They are represented mathematically as numbers that have a remainder of 1 when divided by 2.

2. How can I identify odd numbers between 1 and 1000?

To identify odd numbers between 1 and 1000, look for numbers that end in 1, 3, 5, 7, or 9. The first odd number is 1, and they continue in increments of 2 (1, 3, 5, ..., 999).

3. What is the largest odd number between 1 and 1000?

The largest odd number between 1 and 1000 is 999.

4. Can you provide a list of odd numbers from 1 to 1000?

Certainly! The odd numbers from 1 to 1000 are: 1, 3, 5, 7, 9, 11, 13, 15, ..., 999.

For a complete list, you can generate them by listing every second number starting from 1.

5. How many odd numbers are there between 1 and 1000?

There are 500 odd numbers between 1 and 1000. This is because half of the numbers in that range are odd.

6. Are there any patterns in the sequence of odd numbers?

Yes, odd numbers form an arithmetic sequence where the difference between any two consecutive odd numbers is 2.

7. How do odd numbers relate to even numbers?

Odd numbers are the complementary set of even numbers. Even numbers are integers that can be divided evenly by 2, while odd numbers cannot.

8. What is the sum of all odd numbers from 1 to 1000?

The sum of all odd numbers from 1 to 1000 can be calculated using the formula for the sum of an arithmetic series:
[ S_n = \frac{n}{2} \times (a + l) ]
Where:

• ( n ) = total number of odd numbers (500)

• ( a ) = first term (1)

• ( l ) = last term (999)

Using this information, the sum is: [ S_{500} = \frac{500}{2} \times (1 + 999) = 250 \times 1000 = 250000 ]

9. Can odd numbers be prime numbers?

Yes, some odd numbers are prime numbers. The definition of a prime number is an integer greater than 1 that has no positive divisors other than 1 and itself. Examples of odd prime numbers within 1 to 1000 include 3, 5, 7, 11, and many others.

10. How do odd numbers appear in everyday life?

Odd numbers can be found in various aspects of daily life, from counting objects (e.g., people in a room) to various forms of measurement (quantities, time, etc.). They often appear in games, shopping scenarios, and any situation where non-even distributions are encountered.

Feel free to reach out if you have more questions about odd numbers!

Conclusion

Odd numbers, standing apart from their even counterparts, hold intrigue beyond just their mathematical definition. In mathematics, they form the building blocks of primes, the essential ingredients of number theory. They possess unique properties and exhibit interesting patterns. From identifying them using simple methods to understanding their significance in various mathematical concepts, odd numbers never cease to intrigue us.